Advertisements
Advertisements
प्रश्न
A ray of light coming from the point (1, 2) is reflected at a point A on the x-axis and then passes through the point (5, 3). Find the coordinates of the point A.
उत्तर
Let the incident ray strike x-axis at the point A whose coordinates be (x, 0).
From the figure, the slope of the reflected ray is given by
tan θ = `3/(5 - x)` .....(1)
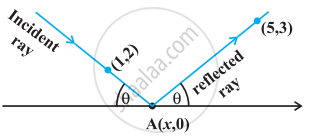
Again, the slope of the incident ray is given by
`tan(pi - theta) = (-2)/(x - 1)` (Why?)
or `- tan theta = (-2)/(x - 1)` ....(2)
Solving (1) and (2), we get
`3/(5 - x) = 2/(x - 1)` or x = `13/5`
Therefore, the required coordinates of the point A are `13/5, 0`.
APPEARS IN
संबंधित प्रश्न
The base of an equilateral triangle with side 2a lies along they y-axis such that the mid point of the base is at the origin. Find vertices of the triangle.
Find the distance between P (x1, y1) and Q (x2, y2) when :
- PQ is parallel to the y-axis,
- PQ is parallel to the x-axis
Without using the Pythagoras theorem, show that the points (4, 4), (3, 5) and (–1, –1) are the vertices of a right angled triangle.
Without using distance formula, show that points (–2, –1), (4, 0), (3, 3) and (–3, 2) are vertices of a parallelogram.
A line passes through (x1, y1) and (h, k). If slope of the line is m, show that k – y1 = m (h – x1).
State whether the two lines in each of the following are parallel, perpendicular or neither.
Through (5, 6) and (2, 3); through (9, −2) and (6, −5)
What can be said regarding a line if its slope is zero ?
What can be said regarding a line if its slope is positive ?
Show that the line joining (2, −3) and (−5, 1) is parallel to the line joining (7, −1) and (0, 3).
Without using the distance formula, show that points (−2, −1), (4, 0), (3, 3) and (−3, 2) are the vertices of a parallelogram.
Find the equation of a straight line with slope − 1/3 and y-intercept − 4.
Find the equations of the bisectors of the angles between the coordinate axes.
Find the equation of the right bisector of the line segment joining the points (3, 4) and (−1, 2).
Find the angles between the following pair of straight lines:
3x − y + 5 = 0 and x − 3y + 1 = 0
If θ is the angle which the straight line joining the points (x1, y1) and (x2, y2) subtends at the origin, prove that \[\tan \theta = \frac{x_2 y_1 - x_1 y_2}{x_1 x_2 + y_1 y_2}\text { and } \cos \theta = \frac{x_1 x_2 + y_1 y_2}{\sqrt{{x_1}^2 + {y_1}^2}\sqrt{{x_2}^2 + {y_2}^2}}\].
Prove that the straight lines (a + b) x + (a − b ) y = 2ab, (a − b) x + (a + b) y = 2ab and x + y = 0 form an isosceles triangle whose vertical angle is 2 tan−1 \[\left( \frac{a}{b} \right)\].
Find k, if the slope of one of the lines given by kx2 + 8xy + y2 = 0 exceeds the slope of the other by 6.
If m1 and m2 are slopes of lines represented by 6x2 - 5xy + y2 = 0, then (m1)3 + (m2)3 = ?
The two lines ax + by = c and a′x + b′y = c′ are perpendicular if ______.
Find the equation of the line passing through the point (5, 2) and perpendicular to the line joining the points (2, 3) and (3, – 1).
Find the angle between the lines y = `(2 - sqrt(3)) (x + 5)` and y = `(2 + sqrt(3))(x - 7)`
Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.
If the equation of the base of an equilateral triangle is x + y = 2 and the vertex is (2, – 1), then find the length of the side of the triangle.
The tangent of angle between the lines whose intercepts on the axes are a, – b and b, – a, respectively, is ______.
The equation of the line through the intersection of the lines 2x – 3y = 0 and 4x – 5y = 2 and
| Column C1 | Column C2 |
| (a) Through the point (2, 1) is | (i) 2x – y = 4 |
| (b) Perpendicular to the line (ii) x + y – 5 = 0 x + 2y + 1 = 0 is |
(ii) x + y – 5 = 0 |
| (c) Parallel to the line (iii) x – y –1 = 0 3x – 4y + 5 = 0 is |
(iii) x – y –1 = 0 |
| (d) Equally inclined to the axes is | (iv) 3x – 4y – 1 = 0 |
The line which passes through the origin and intersect the two lines `(x - 1)/2 = (y + 3)/4 = (z - 5)/3, (x - 4)/2 = (y + 3)/3 = (z - 14)/4`, is ______.
A ray of light coming from the point (1, 2) is reflected at a point A on the x-axis and then passes through the point (5, 3). The co-ordinates of the point A is ______.
The three straight lines ax + by = c, bx + cy = a and cx + ay = b are collinear, if ______.
