Question

A solid cone of base radius 10 cm is cut into two parts through the midpoint of its height, by a plane parallel to its base. Find the ratio of the volumes of the two parts of the cone.

Answer 1

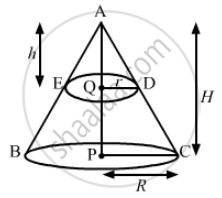
We have,

Radius of solid cone, R = CP = 10 cm,

Let the height of the solid cone be, AP = H,

the radius of the smaller cone, QD = r and

the height of the smaller cone be, AQ = h.

Also, `"AQ"= "AP"/2  "i.e"  "h"="H"/2 or "H = 2h" .......(i)`

Now, in Δ AQD and Δ APC

∠QAD = ∠PAC     (Common angle)

∠AQD = ∠APC = 90°

So, by AA criteria
ΔAQD _˜ ΔAPC

`⇒ ("AQ")/("AP") = ("QD")`

`rArr "h"/"H" = "r"/"R"`   

`rArr "h"/"2h" = r/"R"`    [Using (i)]

`rArr 1/2 = "r"/"R"`

`rArr  "R" = 2"r"`        ..............(ii)

As,

Volume of smaller cone `= 1/3 pi"r"^2"h" `

And, 

Volume of solid cone `= 1/3pi"R"^2H`

`=1/3pi(2"r")^2xx(2"h")`          [Using (i) and (ii)]

`= 8/3pir^2h`

so, Volume frustum = Volume of solid cone - Volume of smaller cone 

`= 8/3pi"r"^2"h" - 1/3pi"r"^2"h"`

`=7/3pi"r"^2"h"`

Now, the ratio of the volumes of the two parts `= "Volume of the smaller cone"/"Volume of the frustum"`

`=(1/3pi"r"^2"h")/(7/3pi"r"^2"h")`

`=1/7`

= 1 : 7

So, the ratio of the volume of the two parts of the cone is 1 : 7.