Advertisements
Advertisements
प्रश्न
A straight line AB is 8 cm long. Draw and describe the locus of a point which is:
- always 4 cm from the line AB.
- equidistant from A and B.
Mark the two points X and Y, which are 4 cm from AB and equidistant from A and B. Describe the figure AXBY.
उत्तर
Steps of construction:

i. Draw a line segment AB equal to 8 cm.
ii. Draw two parallel lines l and m to AB at a distance of 4 cm.
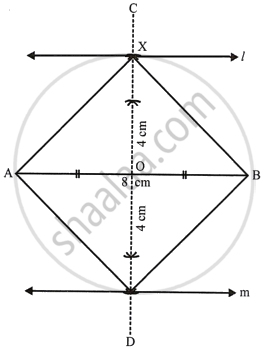
iii. Draw the perpendicular bisector of AB which intersects the parallel lines l and m at X and Y respectively then, X and Y are the required points.
iv. Join AX, AY, BX and BY.
The figure so formed is a square as its diagonals are equal and intersect at 90°.
APPEARS IN
संबंधित प्रश्न
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
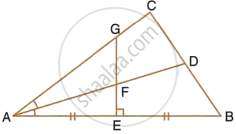
F is equidistant from A and B.
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
In the figure given below, find a point P on CD equidistant from points A and B.

Describe the locus for questions 1 to 13 given below:
1. The locus of a point at a distant 3 cm from a fixed point.
Describe the locus of the moving end of the minute hand of a clock.
Describe the locus of a stone dropped from the top of a tower.
Describe the locus of points at distances less than 3 cm from a given point.
Draw a triangle ABC in which AB = 6 cm, BC = 4.5 cm and AC = 5 cm. Draw and label:
- the locus of the centres of all circles which touch AB and AC,
- the locus of the centres of all the circles of radius 2 cm which touch AB.
Hence, construct the circle of radius 2 cm which touches AB and AC .
In a quadrilateral ABCD, if the perpendicular bisectors of AB and AD meet at P, then prove that BP = DP.
ΔPBC and ΔQBC are two isosceles triangles on the same base BC but on the opposite sides of line BC. Show that PQ bisects BC at right angles.
