Advertisements
Advertisements
प्रश्न
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
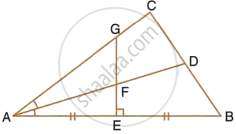
F is equidistant from A and B.
उत्तर

Construction: Join FB and FC
Proof: In ΔAFE and ΔFBE,
AE = EB ...(E is the mid-point of AB)
∠FEA = ∠FEB ...(Each = 90°)
FE = FE ...(Common)
∴ By side Angle side criterion of congruence,
ΔAFE ≅ ΔFBE ...(SAS Postulate)
The corresponding parts of the congruent triangles are congruent.
∴ AF = FB ...(C.P.C.T.)
Hence, F is equidistant from A and B.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.
In triangle LMN, bisectors of interior angles at L and N intersect each other at point A. Prove that:
- Point A is equidistant from all the three sides of the triangle.
- AM bisects angle LMN.
In the figure given below, find a point P on CD equidistant from points A and B.

Describe the locus of the centre of a wheel of a bicycle going straight along a level road.
Describe the locus of the moving end of the minute hand of a clock.
Describe the locus of the door handle, as the door opens.
Describe the locus of points at distances greater than 4 cm from a given point.
In Δ ABC, the perpendicular bisector of AB and AC meet at 0. Prove that O is equidistant from the three vertices. Also, prove that if M is the mid-point of BC then OM meets BC at right angles.
Find the locus of points which are equidistant from three non-collinear points.
Given: ∠BAC, a line intersects the arms of ∠BAC in P and Q. How will you locate a point on line segment PQ, which is equidistant from AB and AC? Does such a point always exist?
