Advertisements
Advertisements
प्रश्न
A travelling wave is produced on a long horizontal string by vibrating an end up and down sinusoidally. The amplitude of vibration is 1⋅0 and the displacement becomes zero 200 times per second. The linear mass density of the string is 0⋅10 kg m−1 and it is kept under a tension of 90 N. (a) Find the speed and the wavelength of the wave. (b) Assume that the wave moves in the positive x-direction and at t = 0, the end x = 0 is at its positive extreme position. Write the wave equation. (c) Find the velocity and acceleration of the particle at x = 50 cm at time t = 10 ms.
उत्तर
Given,
Amplitude of the wave = 1 cm
Frequency of the wave,
\[f = \frac{200}{2} = 100 \text{ Hz }\]
Mass per unit length, m = 0.1 kg/m
Applied tension, T = 90 N
(a) Velocity of the wave is given by
\[v = \sqrt{\frac{T}{m}}\]
Thus, we have:\[v = \sqrt{\left( \frac{90}{0 . 1} \right)} = 30 m/s\]
Now,
\[\text{ Wavelength, } \lambda = \frac{v}{f} = \frac{30}{100} = 0 . 3 m\]
\[ \Rightarrow \lambda = 30 cm\]
(b) At x = 0, displacement is maximum.
Thus, the wave equation is given by
\[y = \left( 1 cm \right)\cos2\pi\left\{ \left( \frac{t}{0 . 01 s} \right) - \left( \frac{x}{30 cm} \right) \right\}\] ...(1)
(c) Using \[\cos\left( - \theta \right) = \cos\theta\]
in equation (1), we get:
\[y = 1\cos2\pi\left( \frac{x}{30} - \frac{t}{0 . 01} \right)\]
\[Velocity, v = \frac{dy}{dt}\]
\[ \Rightarrow v = \left( \frac{2\pi}{0 . 01} \right)\sin2\pi\left\{ \frac{x}{30} - \frac{t}{0 . 01} \right\}\]
And,
Acceleration, \[a = \frac{d\nu}{dt}\]
\[ \Rightarrow a = \left\{ \frac{4 \pi^2}{\left( 0 . 01 \right)^2} \right\}\cos2\pi\left\{ \left( \frac{x}{30} \right) - \left( \frac{t}{0 . 01} \right) \right\}\]
\[\text{ When x = 50 cm, t = 10 ms = 10 \times {10}^{- 3} s .}\]
Now,
\[v = \left( \frac{2\pi}{0 . 01} \right)\sin2\pi\left\{ \left( \frac{5}{3} \right) - \left( \frac{0 . 01}{0 . 01} \right) \right\}\]
\[ = \left( \frac{2\pi}{0 . 01} \right)\sin\left( 2\pi \times \frac{2}{3} \right)\]
\[= - \left( \frac{2\pi}{0 . 01} \right)\sin\frac{4\pi}{3}\]
\[= - 200\pi\sin\frac{\pi}{3}\]
\[= - 200\pi \times \frac{\sqrt{3}}{2}\]
\[= - 544 cm/s\]
\[= - 5 . 4 m/s\]
In magnitude, v = 5.4 m/s.
Similarly,
\[a = \left\{ \frac{4 \pi^2}{\left( 0 . 01 \right)^2} \right\}\cos2\pi\left\{ \left( \frac{5}{3} \right) - 1 \right\}\]
\[ = 4 \pi^2 \times {10}^4 \times \frac{1}{2}\]
\[ \approx 2 \times {10}^5 cm/ s^2 \text{ or 2 km}/ s^2\]
APPEARS IN
संबंधित प्रश्न
A stone dropped from the top of a tower of height 300 m high splashes into the water of a pond near the base of the tower. When is the splash heard at the top given that the speed of sound in air is 340 m s–1? (g= 9.8 m s–2)
Use the formula `v = sqrt((gamma P)/rho)` to explain why the speed of sound in air increases with humidity.
You have learnt that a travelling wave in one dimension is represented by a function y= f (x, t)where x and t must appear in the combination x – v t or x + v t, i.e. y = f (x ± v t). Is the converse true? Examine if the following functions for y can possibly represent a travelling wave:
(a) `(x – vt )^2`
(b) `log [(x + vt)/x_0]`
(c) `1/(x + vt)`
For the travelling harmonic wave
y (x, t) = 2.0 cos 2π (10t – 0.0080x + 0.35)
Where x and y are in cm and t in s. Calculate the phase difference between oscillatory motion of two points separated by a distance of `(3λ)/4`.
A steel rod 100 cm long is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is given to be 2.53 kHz. What is the speed of sound in steel?
Show that the particle speed can never be equal to the wave speed in a sine wave if the amplitude is less than wavelength divided by 2π.
Show that for a wave travelling on a string
\[\frac{y_{max}}{\nu_{max}} = \frac{\nu_{max}}{\alpha_{max}},\]
where the symbols have usual meanings. Can we use componendo and dividendo taught in algebra to write
\[\frac{y_{max} + \nu_{max}}{\nu_{max} - \nu_{max}} = \frac{\nu_{max} + \alpha_{max}}{\nu_{max} - \alpha_{max}}?\]
Two strings A and B, made of same material, are stretched by same tension. The radius of string A is double of the radius of B. A transverse wave travels on A with speed `v_A` and on B with speed `v_B`. The ratio `v_A/v_B` is ______.
Two wave pulses travel in opposite directions on a string and approach each other. The shape of one pulse is inverted with respect to the other.
A wave propagates on a string in the positive x-direction at a velocity \[\nu\] \[t = t_0\] is given by \[g\left( x, t_0 \right) = A \sin \left( x/a \right)\]. Write the wave equation for a general time t.
A wave travelling on a string at a speed of 10 m s−1 causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave? (b) If the displacement of a particle of 1⋅5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant?
Following figure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning for. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. Find the density of the material of the block.
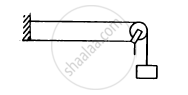
A 2⋅00 m-long rope, having a mass of 80 g, is fixed at one end and is tied to a light string at the other end. The tension in the string is 256 N. (a) Find the frequencies of the fundamental and the first two overtones. (b) Find the wavelength in the fundamental and the first two overtones.
An organ pipe of length 0.4 m is open at both ends. The speed of sound in the air is 340 m/s. The fundamental frequency is ______
A sound wave is passing through air column in the form of compression and rarefaction. In consecutive compressions and rarefactions ______.
A string of mass 2.5 kg is under a tension of 200 N. The length of the stretched string is 20.0 m. If the transverse jerk is struck at one end of the string, the disturbance will reach the other end in ______.
A steel wire has a length of 12 m and a mass of 2.10 kg. What will be the speed of a transverse wave on this wire when a tension of 2.06 × 104N is applied?
The amplitude of wave disturbance propagating in the positive x-direction given is by `1/(1 + x)^2` at time t = 0 and `1/(1 + (x - 2)^2)` at t = 1 s, where x and y are in 2 metres. The shape of wave does not change during the propagation. The velocity of the wave will be ______ m/s.
