Advertisements
Advertisements
Question
A travelling wave is produced on a long horizontal string by vibrating an end up and down sinusoidally. The amplitude of vibration is 1⋅0 and the displacement becomes zero 200 times per second. The linear mass density of the string is 0⋅10 kg m−1 and it is kept under a tension of 90 N. (a) Find the speed and the wavelength of the wave. (b) Assume that the wave moves in the positive x-direction and at t = 0, the end x = 0 is at its positive extreme position. Write the wave equation. (c) Find the velocity and acceleration of the particle at x = 50 cm at time t = 10 ms.
Solution
Given,
Amplitude of the wave = 1 cm
Frequency of the wave,
\[f = \frac{200}{2} = 100 \text{ Hz }\]
Mass per unit length, m = 0.1 kg/m
Applied tension, T = 90 N
(a) Velocity of the wave is given by
\[v = \sqrt{\frac{T}{m}}\]
Thus, we have:\[v = \sqrt{\left( \frac{90}{0 . 1} \right)} = 30 m/s\]
Now,
\[\text{ Wavelength, } \lambda = \frac{v}{f} = \frac{30}{100} = 0 . 3 m\]
\[ \Rightarrow \lambda = 30 cm\]
(b) At x = 0, displacement is maximum.
Thus, the wave equation is given by
\[y = \left( 1 cm \right)\cos2\pi\left\{ \left( \frac{t}{0 . 01 s} \right) - \left( \frac{x}{30 cm} \right) \right\}\] ...(1)
(c) Using \[\cos\left( - \theta \right) = \cos\theta\]
in equation (1), we get:
\[y = 1\cos2\pi\left( \frac{x}{30} - \frac{t}{0 . 01} \right)\]
\[Velocity, v = \frac{dy}{dt}\]
\[ \Rightarrow v = \left( \frac{2\pi}{0 . 01} \right)\sin2\pi\left\{ \frac{x}{30} - \frac{t}{0 . 01} \right\}\]
And,
Acceleration, \[a = \frac{d\nu}{dt}\]
\[ \Rightarrow a = \left\{ \frac{4 \pi^2}{\left( 0 . 01 \right)^2} \right\}\cos2\pi\left\{ \left( \frac{x}{30} \right) - \left( \frac{t}{0 . 01} \right) \right\}\]
\[\text{ When x = 50 cm, t = 10 ms = 10 \times {10}^{- 3} s .}\]
Now,
\[v = \left( \frac{2\pi}{0 . 01} \right)\sin2\pi\left\{ \left( \frac{5}{3} \right) - \left( \frac{0 . 01}{0 . 01} \right) \right\}\]
\[ = \left( \frac{2\pi}{0 . 01} \right)\sin\left( 2\pi \times \frac{2}{3} \right)\]
\[= - \left( \frac{2\pi}{0 . 01} \right)\sin\frac{4\pi}{3}\]
\[= - 200\pi\sin\frac{\pi}{3}\]
\[= - 200\pi \times \frac{\sqrt{3}}{2}\]
\[= - 544 cm/s\]
\[= - 5 . 4 m/s\]
In magnitude, v = 5.4 m/s.
Similarly,
\[a = \left\{ \frac{4 \pi^2}{\left( 0 . 01 \right)^2} \right\}\cos2\pi\left\{ \left( \frac{5}{3} \right) - 1 \right\}\]
\[ = 4 \pi^2 \times {10}^4 \times \frac{1}{2}\]
\[ \approx 2 \times {10}^5 cm/ s^2 \text{ or 2 km}/ s^2\]
APPEARS IN
RELATED QUESTIONS
For the wave described in Exercise 15.8, plot the displacement (y) versus (t) graphs for x = 0, 2 and 4 cm. What are the shapes of these graphs? In which aspects does the oscillatory motion in travelling wave differ from one point to another: amplitude, frequency or phase?
A wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45 Hz. The mass of the wire is 3.5 × 10–2 kg and its linear mass density is 4.0 × 10–2 kg m–1. What is (a) the speed of a transverse wave on the string, and (b) the tension in the string?
A metre-long tube open at one end, with a movable piston at the other end, shows resonance with a fixed frequency source (a tuning fork of frequency 340 Hz) when the tube length is 25.5 cm or 79.3 cm. Estimate the speed of sound in air at the temperature of the experiment. The edge effects may be neglected.
Earthquakes generate sound waves inside the earth. Unlike a gas, the earth can experience both transverse (S) and longitudinal (P) sound waves. Typically the speed of S wave is about 4.0 km s–1, and that of P wave is 8.0 km s–1. A seismograph records P and S waves from an earthquake. The first P wave arrives 4 min before the first S wave. Assuming the waves travel in straight line, at what distance does the earthquake occur?
Two sine waves travel in the same direction in a medium. The amplitude of each wave is A and the phase difference between the two waves is 120°. The resultant amplitude will be
The equation of a wave travelling on a string stretched along the X-axis is given by
\[y = A e {}^- \left( \frac{x}{a} + \frac{t}{T} \right)^2 .\]
(a) Write the dimensions of A, a and T. (b) Find the wave speed. (c) In which direction is the wave travelling? (d) Where is the maximum of the pulse located at t = T? At t = 2 T?
A wave propagates on a string in the positive x-direction at a velocity \[\nu\] \[t = t_0\] is given by \[g\left( x, t_0 \right) = A \sin \left( x/a \right)\]. Write the wave equation for a general time t.
The equation of a wave travelling on a string is \[y = \left( 0 \cdot 10 \text{ mm } \right) \sin\left[ \left( 31 \cdot 4 m^{- 1} \right)x + \left( 314 s^{- 1} \right)t \right]\]
(a) In which direction does the wave travel? (b) Find the wave speed, the wavelength and the frequency of the wave. (c) What is the maximum displacement and the maximum speed of a portion of the string?
A wave travels along the positive x-direction with a speed of 20 m s−1. The amplitude of the wave is 0⋅20 cm and the wavelength 2⋅0 cm. (a) Write the suitable wave equation which describes this wave. (b) What is the displacement and velocity of the particle at x= 2⋅0 cm at time t = 0 according to the wave equation written? Can you get different values of this quantity if the wave equation is written in a different fashion?
Two waves, travelling in the same direction through the same region, have equal frequencies, wavelengths and amplitudes. If the amplitude of each wave is 4 mm and the phase difference between the waves is 90°, what is the resultant amplitude?
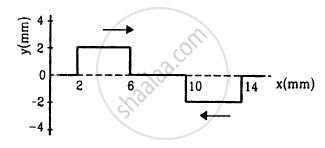
Following figure shows two wave pulses at t = 0 travelling on a string in opposite directions with the same wave speed 50 cm s−1. Sketch the shape of the string at t = 4 ms, 6 ms, 8 ms, and 12 ms.
A 40 cm wire having a mass of 3⋅2 g is stretched between two fixed supports 40⋅05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is 1⋅0 mm2, find its Young modulus.
The string of a guitar is 80 cm long and has a fundamental frequency of 112 Hz. If a guitarist wishes to produce a frequency of 160 Hz, where should the person press the string?
A string 1 m long is fixed at one end. The other end is moved up and down with a frequency of 20 Hz. Due to this, a stationary wave with four complete loops gets produced on the string. Find the speed of the progressive wave which produces the stationary wave.
For the travelling harmonic wave
y (x, t) = 2.0 cos 2π (10t – 0.0080x + 0.35)
Where x and y are in cm and t in s. Calculate the phase difference between oscillatory motion of two points separated by a distance of 0.5 m.
Speed of sound wave in air ______.
Speed of sound waves in a fluid depends upon ______.
- directty on density of the medium.
- square of Bulk modulus of the medium.
- inversly on the square root of density.
- directly on the square root of bulk modulus of the medium.
A wave of frequency υ = 1000 Hz, propagates at a velocity v = 700 m/sec along x-axis. Phase difference at a given point x during a time interval M = 0.5 × 10-3 sec is ______.
Two perfectly identical wires kept under tension are in unison. When the tension in the wire is increased by 1% then on sounding them together 3 beats are heard in 2 seconds. What is the frequency of each wire?
