Advertisements
Advertisements
प्रश्न
A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole, the angle of elevation of the top of the tower is 60° and the angle of depression of the bottom of the tower is 30°. Find:
- the height of the tower, if the height of the pole is 20 m;
- the height of the pole, if the height of the tower is 75 m.
उत्तर
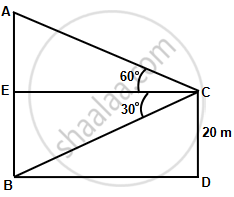
Let AB be the tower and CD be the pole.
Then ∠ACE = 60° and ∠BCE = 30°.
i. In ΔBEC,
`(BE)/(EC) = tan 30^circ`
`=> 20/(EC) = 1/sqrt(3)`
`=> EC = 20sqrt(3) m`
In ΔAEC,
`(AE)/(EC) = tan 60^circ`
`=> AE = 20sqrt(3) xx sqrt(3) = 60 m `
∴ Height of the tower = AB
= AE + EB
= (60 + 20)
= 80 m
ii. Let height of the pole be x m
∴ CD = BE = x
In ΔBEC,
`(BE)/(EC) = tan 30^circ`
`=> EC = sqrt(3)x`
In ΔAEC,
`(AE)/(EC) = tan 60^circ`
`=> (75 - x)/(EC) = sqrt(3)`
`=>` 75 – x = 3x
∴ `x = 75/4 = 18.75 m`
∴ Height of the pole is 18.75 m.
APPEARS IN
संबंधित प्रश्न
The angles of elevation of the top of a tower from two points on the ground at distances a and b metres from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is `sqrt(ab)` metre.
Due to a heavy storm, a part of a banyan tree broke without separating from the main. The top of the tree touched the ground 15 m from the base making an angle of 45° with the ground. Calculate the height of the tree before it was broken.
Find the angle of depression from the top of a 140m high pillar of a milestone on the ground at a distance of 200m from the foot of the pillar.
An observer, 1.5m tall, is 28.5m away from a tower 30m high. Determine the angle of elevation of the top of the tower from his eye.
From the top of a light house 96m high, the angles of depression of two ships in the river and at the same level as the base of the light house and on the same side of it, are α and β. If tan α = `1/4` and tan β = `1/7`, find the distance between the ships.
A man on the deck of a ship is 10 m above the water level. He observes that the angle of elevation of the top of a diff is 45° and the angle of depression of the base is 30°. Find the distance of the diff from the ship and the height of the cliff.
A man on the top of a tower observes that a car is moving directly at a uniform speed towards it. If it takes 720 seconds for the angle of depression to change from 30° to 45°, how soon will the car reach the observation tower?
From an aeroplane vertically above a straight horizontal road, the angles of depression of two consecutive milestone on opposite sides of the aeroplane are observed to be α, and β. Show that the height in miles of aeroplane above the road is `(tanα tanβ)/(tanα + tanβ)`.
A man on the top of vertical observation tower observes a car moving at a uniform speed coming directly towards it. If it takes 12 minutes for the angle of depression to change from 30° to 45°, how soon after this will the car reach the observation tower? (Give your answer correct to nearest seconds).
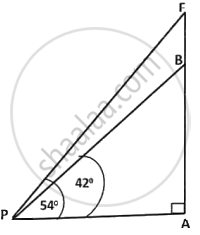
A vertical tower standing on a horizontal plane is surmounted by a vertical flagstaff. At a point 100 m away from the foot of the tower, the angle of elevation of the top and bottom of the flagstaff are 54° and 42° respectively. Find the height of the flagstaff. Give your answer correct to nearest metre.