Advertisements
Advertisements
प्रश्न
ABCD is a trapezium in which AB || DC. P and Q are points on sides AD and BC such that PQ || AB. If PD = 18, BQ = 35 and QC = 15, find AD.
उत्तर
In trapezium ABCD, AB || DC. P and Q are points on sides AD and BC such that PQ || AB.
Join AC. Suppose AC intersects PQ in O.
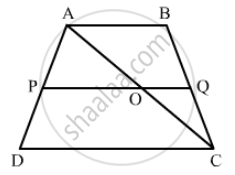
In Δ ACD , OP || CD
`∴ (AP)/(PD)=(AO)/(OC)`.......(1) (BPT)
`In Δ Abc , OQ||AB`
`∴ (BQ)/(QC)=(AO)/(OC)`.......(2) (BPT)
From (1) and (2), we get
`(AP)/(DP)=(BQ)/(QC)`
`(AP)/(18)=(35)/(15)`
`AP=(35xx18)/(5xx3)`
`AP=(7xx5xx3xx6)/(5xx3)`
`AP=(7xxcancel5xxcancel3xx6)/(cancel5xxcancel3)`
`AP = 42`
`AD=AP+PD`
`AD=42+18`
`AD=60`
Hence, the value of AD is 60 .
APPEARS IN
संबंधित प्रश्न
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2m/sec. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AB = 10.8cm, AD = 6.3cm, AC = 9.6cm and EC = 4cm.

In ∆ABC, points P and Q are on CA and CB, respectively such that CA = 16 cm, CP = 10 cm, CB = 30 cm and CQ = 25 cm. Is PQ || AB?
In ∆ABC, P and Q are points on sides AB and AC respectively such that PQ || BC. If AP = 4 cm, PB = 6 cm and PQ = 3 cm, determine BC.
In the given figure, l || m
(i) Name three pairs of similar triangles with proper correspondence; write similarities.
(ii) Prove that

Corresponding sides of two triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, determine the area of the larger triangle.
A point D is on the side BC of an equilateral triangle ABC such that\[DC = \frac{1}{4}BC\]. Prove that AD2 = 13 CD2.
In ∆ABC, ∠ABC = 135°. Prove that AC2 = AB2 + BC2 + 4 ar (∆ABC)
In a quadrilateral ABCD, ∠B = 90°. If AD2 = AB2 + BC2 + CD2 then prove that ∠ACD = 90°.
In triangles ABC and DEF, ∠A = ∠E = 40°, AB : ED = AC : EF and ∠F = 65°, then ∠B =
