Advertisements
Advertisements
प्रश्न
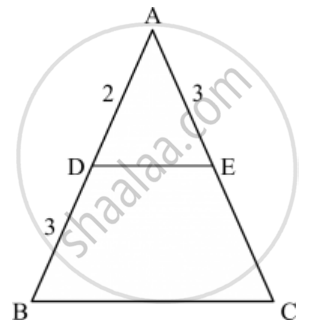
In ∆ABC, D and E are points on sides AB and AC respectively such that AD ✕ EC = AE ✕ DB. Prove that DE || BC.
उत्तर
Given: In Δ ABC, D and E are points on sides AB and AC such that `ADxxECxxAExxDB`
To Prove: DE||BC
Proof:
Since `ADxxECxxAExxDB`
`⇒ (DB)/(AD)=(EC)/(AE)`
`⇒ (DB)/(AD)+1=(EC)/(AE)+1`
`⇒ (DB+AD)/(AD)=(EC+AE)/(AE)`
`⇒ (AB)/(AD)=(AC)/(AE)`
∴ DE || BC (Converse of basic proportionality theorem)
APPEARS IN
संबंधित प्रश्न
In the below figure, If AB || CD, find the value of x.

A vertical stick of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AD = 7.2cm, AE = 6.4cm, AB = 12cm and AC = 10cm.
In a ΔABC, AD is the bisector of ∠A.
If AB = 5.6cm, AC = 4cm and DC = 3cm, find BC.

State SAS similarity criterion.
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, what is the length of the longest side of the smaller triangle?
In the given figure, DE || BC in ∆ABC such that BC = 8 cm, AB = 6 cm and DA = 1.5 cm. Find DE.

In a ∆ABC, AD is the bisector of ∠BAC. If AB = 8 cm, BD = 6 cm and DC = 3 cm. Find AC
In an equilateral triangle ABC if AD ⊥ BC, then AD2 =
In the given figure, if ∠ADE = ∠ABC, then CE =