Advertisements
Advertisements
प्रश्न
Activation energy of a chemical reaction can be determined by ______.
पर्याय
determining the rate constant at standard temperature.
determining the rate constants at two temperatures.
determining probability of collision.
using catalyst.
उत्तर
Activation energy of a chemical reaction can be determined by determining the rate constants at two temperatures.
Explanation:
`log k_2/k_1 = E_a/(2.303R)(1/T_1 - 1/T_2)`
Where, Ea = activation energy
T2 = higher temperature
T1 = lower temperature
k1 = rate constant at temperature T1
k2 = rate constant at temperature T2
This equation is known as Arrhenius equation.
APPEARS IN
संबंधित प्रश्न
The rate constant of a first order reaction increases from 4 × 10−2 to 8 × 10−2 when the temperature changes from 27°C to 37°C. Calculate the energy of activation (Ea). (log 2 = 0.301, log 3 = 0.4771, log 4 = 0.6021)
The rate constant of a first order reaction increases from 2 × 10−2 to 4 × 10−2 when the temperature changes from 300 K to 310 K. Calculate the energy of activation (Ea).
(log 2 = 0.301, log 3 = 0.4771, log 4 = 0.6021)
The decomposition of a hydrocarbon has value of rate constant as 2.5×104s-1 At 27° what temperature would rate constant be 7.5×104 × 3 s-1if energy of activation is 19.147 × 103 J mol-1 ?
The rate of chemical reaction becomes double for every 10° rise in temperature because of ____________.
Consider figure and mark the correct option.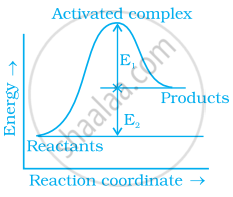
The activation energy in a chemical reaction is defined as ______.
The activation energy in a chemical reaction is defined as ______.
Arrhenius equation can be represented graphically as follows:

The (i) intercept and (ii) slope of the graph are:
An exothermic reaction X → Y has an activation energy 30 kJ mol-1. If energy change ΔE during the reaction is - 20 kJ, then the activation energy for the reverse reaction in kJ is ______.
A first-order reaction is 50% complete in 30 minutes at 300 K and in 10 minutes at 320 K. Calculate activation energy (Ea) for the reaction. [R = 8.314 J K−1 mol−1]
[Given: log 2 = 0.3010, log 3 = 0.4771, log 4 = 0.6021]
