Advertisements
Advertisements
प्रश्न
An arbitrary surface encloses a dipole. What is the electric flux through this surface?
उत्तर
According to Gauss' law, the electric flux through an enclosed surface is given by `oint_s vecE * dvecS = q_(enclosed)/ε_0`.
The net charge on a dipole is given by –q + q = 0, hence `q_(enclosed)` = 0
Hence the electric flux through a surface enclosing a dipole = `(-q + q)/ε_0 = q/ε_0` = 0
APPEARS IN
संबंधित प्रश्न
State and explain Gauss’s law.
A charge ‘q’ is placed at the centre of a cube of side l. What is the electric flux passing through each face of the cube?
Draw a graph of electric field E(r) with distance r from the centre of the shell for 0 ≤ r ≤ ∞.
Gaussian surface cannot pass through discrete charge because ____________.
q1, q2, q3 and q4 are point charges located at points as shown in the figure and S is a spherical gaussian surface of radius R. Which of the following is true according to the Gauss' law?

Five charges q1, q2, q3, q4, and q5 are fixed at their positions as shown in figure. S is a Gaussian surface. The Gauss’s law is given by `oint_s E.ds = q/ε_0`
Which of the following statements is correct?
If `oint_s` E.dS = 0 over a surface, then ______.
- the electric field inside the surface and on it is zero.
- the electric field inside the surface is necessarily uniform.
- the number of flux lines entering the surface must be equal to the number of flux lines leaving it.
- all charges must necessarily be outside the surface.
Consider a region inside which there are various types of charges but the total charge is zero. At points outside the region
- the electric field is necessarily zero.
- the electric field is due to the dipole moment of the charge distribution only.
- the dominant electric field is `∞ 1/r^3`, for large r, where r is the distance from a origin in this region.
- the work done to move a charged particle along a closed path, away from the region, will be zero.
Refer to the arrangement of charges in figure and a Gaussian surface of radius R with Q at the centre. Then
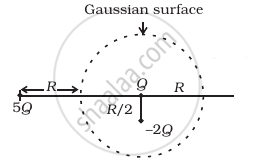
- total flux through the surface of the sphere is `(-Q)/ε_0`.
- field on the surface of the sphere is `(-Q)/(4 piε_0 R^2)`.
- flux through the surface of sphere due to 5Q is zero.
- field on the surface of sphere due to –2Q is same everywhere.
A charge Q is placed at the centre of a cube. The electric flux through one of its faces is ______.
