Advertisements
Advertisements
प्रश्न
Derive the equation for acceptance angle and numerical aperture, of optical fiber.
उत्तर
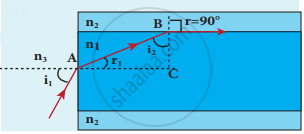
- To ensure the critical angle incident in the core-cladding boundary inside the optical fibre, the light should be incident at a certain angle at the end of the optical fiber while entering into it. This angle is called an acceptance angle.
acceptance angle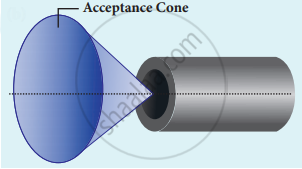
acceptance cone - By Snell’s law
n3 sin ia = n1 sin ra
To have the internal reflection inside optical fibre,
n1 sin i1 = n2 sin 90°
n1 sin ic = n2 sin 90° = 1
∴ sin ic = `"n"_2/"n"_1` - From the right angle triangle AABC,
ic = 90° – ra
Now, equation becomes
sin (90° – ra) = `"n"_2/"n"_1` - Using trigonometry,
cos ra = `"n"_2/"n"_1`
sin ra = `sqrt(1 - cos^2 "r"_"a")`
Substituting for cos ra
sin ra = `sqrt(1 - ("n"_2/"n"_1)^2) = sqrt(("n"_1^2 - "n"_2^2)/"n"_1^2)`
Substituting this in equation (1)
n3 sin ia = `"n"_1 sqrt(("n"_1^2 - "n"_2^2)/"n"_1^2) = sqrt("n"_1^2 - "n"_2^2)`
On further simplification,
sin ia = `sqrt(("n"_1^2 - "n"_2^2)/"n"_3)` or sin ia = `sqrt(("n"_1^2 - "n"_2^2)/"n"_3)`
`"i"_"a" = sin^-1 (sqrt(("n"_1^2 - "n"_2^2)/"n"_3))`
If outer medium is air, then n3 = 1. The acceptance angle ia becomes,
`"i"_"a" = sin^-1 (sqrt("n"_1^2 - "n"_2^2))` - Light can have any angle of incidence from o to ia with the normal at the end of the optical fibre forming a conical shape called acceptance cone.
The term (n3 sin ia) is called numerical aperture NA of the optical fibre
NA = n3 sin ia = `sqrt("n"_1^2 - "n"_2^2)` - 6. If outer medium is air, then n3 = 1. The numeric aperture NA becomes,
NA = sin ia = `sqrt("n"_1^2 - "n"_2^2)`
APPEARS IN
संबंधित प्रश्न
Light of wavelength 5000 Å propagating in air gets partly reflected from the surface of water. How will the wavelengths and frequencies of the reflected and refracted light be affected?
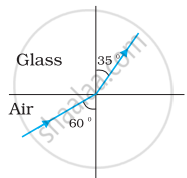
Figures (a) and (b) show the refraction of a ray in air incident at 60° with the normal to a glass-air and water-air interface, respectively. Predict the angle of refraction in the glass when the angle of incidence in water is 45° with the normal to a water-glass interface [Figure (c)].
 |
 |
 |
| (a) | (b) | (c) |
The refractive index of diamond is much greater than that of ordinary glass. Is this fact of some use to a diamond cutter?
For the same value of angle of incidence, the angles of refraction in three media A, B and C are 15°, 25° and 35° respectively. In which medium would the velocity of light be minimum?
A pole of length 1.00 m stands half dipped in a swimming pool with water level 50.0 cm higher than the bed. The refractive index of water is 1.33 and sunlight is coming at an angle of 45° with the vertical. Find the length of the shadow of the pole on the bed.
An object P is focussed by a microscope M. A glass slab of thickness 2.1 cm is introduced between P and M. If the refractive index of the slab is 1.5, by what distance should the microscope be shifted to focus the object again?
What is optical path? Obtain the equation for optical path of a medium of thickness d and refractive index n.
An object is immersed in a fluid of refractive index 'µ'. In order that the object becomes invisible when observed from outside, it should ______.
There are certain material developed in laboratories which have a negative refractive index (Figure). A ray incident from air (medium 1) into such a medium (medium 2) shall follow a path given by.
A convex lens made of material of refractive index 1.5 and having a focal length of 10 cm is immersed in a liquid of refractive index 3.0. The lens will behave as ______.
