Advertisements
Advertisements
प्रश्न
Evaluate without using trigonometric tables.
`2((tan 35^@)/(cot 55^@))^2 + ((cot 55^@)/(tan 35^@)) - 3((sec 40^@)/(cosec 50^@))`
उत्तर
`2((tan 35^@)/(cot 55^@))^2 + ((cot 55^@)/tan 35^@)^2 - ((sec 40^@)/(cosec 50^@))`
`= 2((tan (90^@ - 55^@))/cot 55^@)^2 + ((cot(90^@ - 35^@))/tan 35^@)^2 - 3((sec (90^@ - 50^@))/(cosec 50^@))`
`= 2((cot 55^@)/(cot 55^@))^2 + ((tan 35^@)/(tan 35^@))^2 - 3((cosec 50^@)/(cosec 50^@))` [∵ `tan (90^@ - theta) = cot theta` `cot(90^@ - theta) = tan theta` ` sec(90^@ - theta) = cosec theta`]
`= 2(1)^2 + (1)^2 - 3(1)`
= 2 + 1 - 3
= 0
APPEARS IN
संबंधित प्रश्न
The horizontal distance between two towers is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the second tower is 30° and 24° respectively.
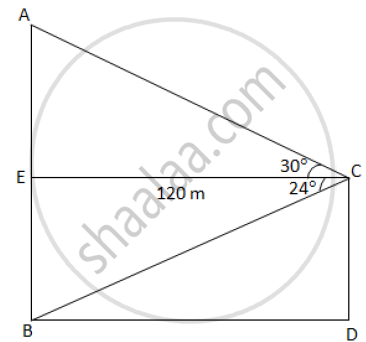
Find the height of the two towers. Give your answer correct to 3 significant figures
In the figure given, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to 30o and 60o respectively. Find:
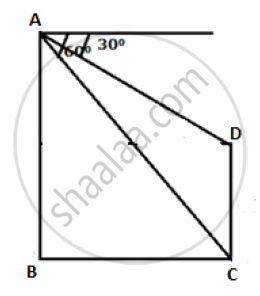
1) The horizontal distance between AB and CD.
2) The height of the lamp post.
Prove the following identities:
sin4A – cos4A = 2sin2A – 1
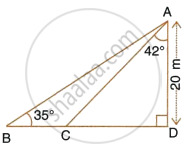
Calculate BC.
An observer point for ships moving in the sea 500m above the sea level. The person manning this point observes the angle of depression of twc boats as 45° and 30°. Find the distance between the boats when they are on the same side of the observation point and when they are on opposite sides of the observation point.
A ladder rests against a wall at an angle a, to the horizontal. Its foot is pulled away from the wall through a distance 'a', so that it slides a distance 'b' down the wall making an angle β with the horizontal. Show that `"a"/"b" = (cosα - cosβ)/(sinβ - sinα).`
In figures, find the length CF.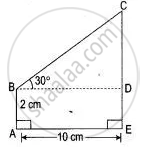
From the top of a lighthouse 100 m high the angles of depression of two ships on opposite sides of it are 48° and 36° respectively. Find the distance between the two ships to the nearest metre.

A man on the top of vertical observation tower observes a car moving at a uniform speed coming directly towards it. If it takes 12 minutes for the angle of depression to change from 30° to 45°, how soon after this will the car reach the observation tower? (Give your answer correct to nearest seconds).
From two points A and B on the same side of a building, the angles of elevation of the top of the building are 30° and 60° respectively. If the height of the building is 10 m, find the distance between A and B correct to two decimal places.
