Advertisements
Advertisements
प्रश्न
From two points A and B on the same side of a building, the angles of elevation of the top of the building are 30° and 60° respectively. If the height of the building is 10 m, find the distance between A and B correct to two decimal places.
उत्तर
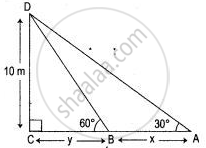
Let CD is building A and B are two given points using horizontally on the same side of building.
In Δ DBC,
tan 60° = `"DC"/"CB"`
√3 = `10/y` .....(1)
In Δ DCA,
tan 30° = `"DC"/"CA"`
`1/(√3) = 10/(x + y)` .....(2)
From (1), put y = `10/sqrt3` in (2), we get
`1/sqrt3 = 10/(x + 10/sqrt3)`
`1/sqrt3 = (10sqrt3)/(sqrt3x + 10)`
30 = √3x + 10
x = `20/sqrt3`
x = 11.55 m.
Hence, distance between two points A and B is 11.55 m.
APPEARS IN
संबंधित प्रश्न
A conical tent is to accommodate 77 persons. Each person must have 16 m3 of air to breathe. Given the radius of the tent as 7 m, find the height of the tent and also its curved surface area.
As observed from the top of a 80 m tall lighthouse, the angles of depression of two ships, on the same side of a light house in a horizontal line with its base, are 30° and 40° respectively. Find the distance between the two ships. Give your answer corrected to the nearest metre.
Find the length of the shadow cast by a tree 60 m high when the sun's altitude is `30^circ`.
A 1.4m tall boy stands at a point 50m away from a tower and observes the angle of elevation of the top of the tower to be 60°. Find the height of the tower.
Two persons standing on opposite sides of a tower observe the angles of elevation of the top of the tower to be 60° and 50° respectively. Find the distance between them, if the height of the tower is 80m.
An aeroplane at an altitude of 200 m observes the angles of depression of opposite points on the two banks of a river to be 45° and 60°. Find the width of the river.
A man on the deck of a ship is 10 m above the water level. He observes that the angle of elevation of the top of a diff is 45° and the angle of depression of the base is 30°. Find the distance of the diff from the ship and the height of the cliff.
The length of the shadow of a statue increases by 8m, when the latitude of the sun changes from 45° to 30°. Calculate the height of the tower.
If the angle of elevation of a cloud from a point h m above a lake is α, and the angle of depression of its reflection in the lake be β, prove that distance of the cloud from the point of observation is `("2h"secα)/(tanα - tanβ)`.
A round balloon of radius 'a' subtends an angle θ at the eye of the observer while the angle of elevation of its centre is Φ. Prove that the height of the centre of the balloon is a sin Φ cosec `θ/2`.
