Advertisements
Advertisements
प्रश्न
Find the coordinates of the points which divide the line segment joining A (−2, 2) and B (2, 8) into four equal parts.
उत्तर १

From the figure, it can be observed that points P, Q, R are dividing the line segment in a ratio 1 : 3, 1 : 1, 3 : 1 respectively.
Coordinates of P =
`((1xx2+3xx(-2))/(1+3),(1xx8+3xx2)/(1+3))`
= `(-1, 7/2)`
Coordinates of Q = `((2+(-2))/2, (2+8)/2)`
= (0, 5)
Coordinates of R = `((3xx2+1xx(-2))/(3+1), (3xx8+1xx2)/(3+1))`
= `(1, 13/2)`
उत्तर २
The coordinates of the midpoint (xm, ym) between two points (x1, y1) and (x2, y2) is given by,
`(x_m,y_m) = ((x_1 + x_2)/2)"," ((y_1 + y_2)/2)`
Here we are supposed to find the points which divide the line joining A(−2, 2) and B(2, 8) into 4 equal parts.
We shall first find the midpoint M(x, y) of these two points since this point will divide the line into two equal parts.
`(x_m, y_m) = ((-2+2)/2)","((2+ 8)/2)`
`(x_m, y_m) = (0,5)`
So the point M(0, 5) splits this line into two equal parts.
Now, we need to find the midpoint of A(−2, 2) and M(0, 5) separately and the midpoint of B(2, 8) and M(0, 5). These two points along with M(0, 5) split the line joining the original two points into four equal parts.
Let M1(e, d) be the midpoint of A(−2, 2) and M(0, 5).
`(e,d) = ((-2 + 0)/2)"," ((2 +5)/2)`
`(e,d) = (-1, 7/2)`
Now let M2(g, h) be the midpoint of B(2, 8) and M(0, 5).
`(g,h) = ((2 +0)/2)","((8 + 5)/2)`
`(g,h) = (1, 13/2)`
Hence, the co-ordinates of the points which divide the line joining the two given points are `(-1, 7/2)`, (0, 5) and `(1, 13/2)`.
APPEARS IN
संबंधित प्रश्न
Find the coordinates of points which trisect the line segment joining (1, –2) and (–3, 4)
Prove that the points (–2, –1), (1, 0), (4, 3) and (1, 2) are the vertices of a parallelogram. Is it a rectangle ?
If A and B are (−2, −2) and (2, −4), respectively, find the coordinates of P such that `"AP" = 3/7 "AB"` and P lies on the line segment AB.
If a vertex of a triangle be (1, 1) and the middle points of the sides through it be (-2,-3) and (5 2) find the other vertices.
The line joining the points A (–3, –10) and B (–2, 6) is divided by the point P such that `(PB)/(AB) = 1/5`. Find the co-ordinates of P.
A(20, 0) and B(10, –20) are two fixed points. Find the co-ordinates of the point P in AB such that : 3PB = AB. Also, find the co-ordinates of some other point Q in AB such that : AB = 6 AQ.
Find the coordinate of a point P which divides the line segment joining :
A(-8, -5) and B (7, 10) in the ratio 2:3.
The line joining P (-5, 6) and Q (3, 2) intersects the y-axis at R. PM and QN are perpendiculars from P and Q on the x-axis. Find the ratio PR: RQ.
Q is a point on the line segment AB. The coordinates of A and B are (2, 7) and (7, 12) along the line AB so that AQ = 4BQ. Find the coordinates of Q.
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
In what ratio is the line joining (2, -1) and (-5, 6) divided by the y axis ?
Using section formula, show that the points A(7, −5), B(9, −3) and C(13, 1) are collinear
If point P divides segment AB in the ratio 1 : 3 where A(– 5, 3) and B(3, – 5), then the coordinates of P are ______
The points (-5, 1), (1, p) and (4, -2) are collinear if the value of p is ______.
Point P(5, –3) is one of the two points of trisection of the line segment joining the points A(7, –2) and B(1, –5).
In what ratio does the x-axis divide the line segment joining the points (– 4, – 6) and (–1, 7)? Find the coordinates of the point of division.
Find the ratio in which the line 2x + 3y – 5 = 0 divides the line segment joining the points (8, –9) and (2, 1). Also find the coordinates of the point of division.
Complete the following activity to find the coordinates of point P which divides seg AB in the ratio 3:1 where A(4, – 3) and B(8, 5).
Activity: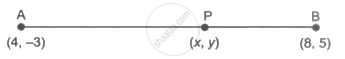
∴ By section formula,
∴ x = `("m"x_2 + "n"x_1)/square`,
∴ x = `(3 xx 8 + 1 xx 4)/(3 + 1)`,
= `(square + 4)/4`,
∴ x = `square`,
∴ y = `square/("m" + "n")`
∴ y = `(3 xx 5 + 1 xx (-3))/(3 + 1)`
= `(square - 3)/4`
∴ y = `square`
A line intersects y-axis and x-axis at point P and Q, respectively. If R(2, 5) is the mid-point of line segment PQ, them find the coordinates of P and Q.
What is the ratio in which the line segment joining (2, -3) and (5, 6) is divided by x-axis?
