Question

For an LCR circuit driven at frequency ω, the equation reads

`L (di)/(dt) + Ri + q/C = v_i = v_m` sin ωt

1. Multiply the equation by i and simplify where possible.
2. Interpret each term physically.
3. Cast the equation in the form of a conservation of energy statement.
4. Integrate the equation over one cycle to find that the phase difference between v and i must be acute.

Answer 1

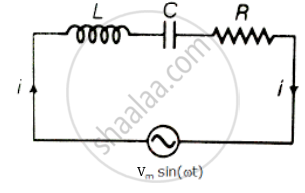
Consider L-C-R series circuit with AC supply

V = V_m sin ωt

Applying voltage Kirchhoff's law over the circuit

∴ V_L + V_C + V_R = Vm sin ωt

L = `(di)/(dt) + Ri + q/c` = V_i = V_m sin ωt

Multiply the above equation by i on both the sides.

L = `(di)/(dt) + Ri + q/c` = V_i = V_m sin ωt

Multiply the above equation by `1/2` on both sides

`1/2 Li (di)/(dt) + i q/(2C) + (i^2R)/2 = 1/2` V_mi sin ωt `(∵ i = (dq)/(dt))`

`(d(1/2 Li^2))/(dt) + 1/(2C) (dq^2)/(dt) + (i^2R)/2 = 1/2` V_mi sin ωt  .....(I)

(i) `(d(1/2  li^2))/(dt)` Represents the rate of change of potential energy in inductance L.

(ii) `d/(dt) q^2/(2C)` represents energy stored in dt time in the capacitor.

(iii) i²R represents joules heating loss.

(iv) `1/2 V_m i` sin ωt is the rate at which driving force pours in energy. It goes into ohmic loss and increase of stored energy in capacitor and inductor.

Vi = rate at which driving force pours in energy. It goes into (i) ohmic loss and (ii) increase of stored energy.

Hence equation (ii) is in the form of conservation of energy statement. Integrating both sides of equation. (ii) with respect to time over one full cycle (0 → T) we may write

`int_0^T  d/(dt) (1/2 Li^2 + q^2/(2C)) dt + int_0^T  Ri^2 dt = int_0^T  Vi  dt`

⇒ 0 + (+ ve) = `int_0^T Vi  dt`

⇒ `int_0^T  Vi  dt  > 0` if phase difference between V and i is a constant and acute angle.