Advertisements
Advertisements
प्रश्न
Four cows are tethered at the four corners of a square field of side 50 m such that each can graze the maximum unshared area. What area will be left ungrazed?
उत्तर
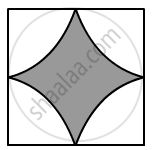
Each cow can graze a region that cannot be accessed by other cows.
∴ Radius of the region grazed by each cow `=50/2 = 25 "m"`
Area that each cow grazes`=1/4xxpixx"r"^2`
`= 1/4xx3.14xx25xx25`
= 490.625 cm2
Total area grazed = 4 × 490.625 = 1963.49 m2
Area of the square = (Side)2
= 502
= 2500 cm2
Now,
Area left ungrazed= Area of the square - Grazed area
= 2500 - 1963.49 = 536.51 m2
APPEARS IN
संबंधित प्रश्न
Area of a sector of angle p (in degrees) of a circle with radius R is ______.
A sector of 56° cut out from a circle contains area of 4.4 cm2. Find the radius of the circle

Area of a sector of central angle 200° of a circle is 770 cm2. Find the length of the corresponding arc of this sector.
The radius of a circle is 17.5 cm. Find the area of the sector enclosed by two radii and an arc 44 cm in length.
In following fig., ABCD is a square. A cirde is drawn with centre A so that it cuts AB and AD at Mand N respectively. Prove that Δ DAM ≅ Δ .BAN.
Find the area of the shaded region where ABC is a quadrant of radius 5cm and a semicircle is drawn with BC as diameter.

If the length of an arc of a circle of radius r is equal to that of an arc of a circle of radius 2r, then the angle of the corresponding sector of the first circle is double the angle of the corresponding sector of the other circle. Is this statement false? Why?
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
With vertices A, B and C of ΔABC as centres, arcs are drawn with radii 14 cm and the three portions of the triangle so obtained are removed. Find the total area removed from the triangle.
