Advertisements
Advertisements
प्रश्न
From a point T outside a circle of centre O, tangents TP and TQ are drawn to the circle. Prove that OT is the right bisector of line segment PQ.
उत्तर
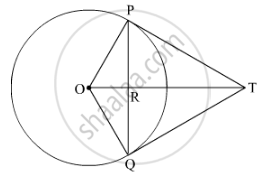
TP and TQ are tangents drawn from an external point T to the circle. O is the centre of the circle.
Suppose OT intersect PQ at point R.
In ∆OPT and ∆OQT,
OP = OQ (Radii of the circle)
TP = TQ (Lengths of tangents drawn from an external point to a circle are equal.)
OT = OT (Common sides)
∴ ∆OPT ≅ ∆OQT (By SSS congruence rule)
So, ∠PTO = ∠QTO (By CPCT) .....(1)
Now, in ∆PRT and ∆QRT,
TP = TQ (Lengths of tangents drawn from an external point to a circle are equal.)
∠PTO = ∠QTO [From (1)]
RT = RT (Common sides)
∴ ∆PRT ≅ ∆QRT (By SAS congruence rule)
So, PR = QR .....(2) (By CPCT)
And, ∠PRT = ∠QRT (By CPCT)
Now,
∠PRT + ∠QRT = 180° (Linear pair)
⇒ 2∠PRT = 180°
⇒ ∠PRT = 90°
∴ ∠PRT = ∠QRT = 90° .....(3)
From (2) and (3), we can conclude that
OT is the right bisector of the line segment PQ.
APPEARS IN
संबंधित प्रश्न
In fig. XP and XQ are tangents from X to the circle with centre O. R is a point on the circle. Prove that, XA + AR = XB + BR.
Fill in the blanks:
A circle divides the plane, on which it lies, in __________ parts.
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO~Δ ABC.

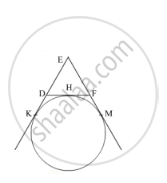
In Fig 2, a circle touches the side DF of ΔEDF at H and touches ED and EF produced at K and M respectively. If EK = 9 cm, then the perimeter of ΔEDF (in cm) is:
AB and CD are common tangents to two circles of equal radii. Prove that AB = CD.
AB is a chord of a circle with centre O , AOC is a diameter and AT is the tangent at A as shown in Fig . 10.70. Prove that \[\angle\]BAT = \[\angle\] ACB.
In following fig. ABC is an equilateral triangle . A circle is drawn with centre A so that ot cuts AB and AC at M and N respectively. Prove that BN = CM.

In the figure, a circle with center P touches the semicircle at points Q and C having center O. If diameter AB = 10, AC = 6, then find the radius x of the smaller circle.
In the figure, O is the centre of a circle, AB is a chord, and AT is the tangent at A. If ∠AOB = 100°, then ∠BAT is equal to ______

A quadrilateral ABCD is inscribed in a circle such that AB is a diameter and ∠ADC = 130º. Find ∠BAC.
