Advertisements
Advertisements
प्रश्न
If A = 600 and B = 300, verify that:
(iii) tan (A-B) = `(tan A-tanB)/(1+tan A tan B)`
उत्तर
tan (A – B) = tan 600 =` 1/sqrt(3)`
`(tan A - tan B)/(1+ tan A tan B) = (tan 60^0 - tan 30^60)/(1+tan60^0tan30^0) = (sqrt(3)-(1/sqrt(3))) /(1+(sqrt(3) xx1/sqrt(3))` = `1/2 xx(3-1)/sqrt(3) = 3/sqrt(3)`
∴ `tan (A-B) = (tan A- tan B)/(1+ tan A tan B)`
APPEARS IN
संबंधित प्रश्न
If A = B = 60°, verify that sin (A − B) = sin A cos B − cos A sin B
If sin 3θ = cos (θ – 6°) where 3θ and θ − 6° are acute angles, find the value of θ.
If cos θ = `3/5` , show that `((sin theta - cot theta ))/(2tan theta)=3/160`
In ΔABC , ∠C = 90° ∠ABC = θ° BC = 21 units . and AB= 29 units. Show thaT `(cos^2 theta - sin^2 theta)=41/841`
If sin (A+B) = sin A cos B + cos A sin B and cos (A-B) = cos A cos B + sin A sin B
(i) sin (750)
(ii) cos (150)
Given: cos A = `( 5 )/ ( 13 )`
Evaluate:
- `(sin "A "–cot "A") / (2 tan "A")`
- `cot "A" + 1/cos"A"`
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
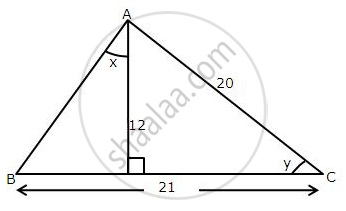
Use the information given in the following figure to evaluate:
`(10)/sin x + (6)/sin y – 6 cot y`.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of cos y
