Advertisements
Advertisements
प्रश्न
If AB = 12 cm, BC = 16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is ______.
पर्याय
6 cm
8 cm
10 cm
12 cm
उत्तर
If AB = 12 cm, BC = 16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is 10 cm.
Explanation:
Given in the question, AB = 12 cm and BC = 16 cm.
In a circle, BC ⊥ AB. So, that means AC will be a diameter of circle.
Now, by using Pythagoras theorem in right-angled triangle ABC.
AC2 = AB2 + BC2
AC2 = (12)2 + (16)2
AC2 = 144 + 256
AC2 = 400
AC = 20 cm
So, radius of circle = `1/2 xx AC`
= `1/2 xx 20`
= 10 cm
Therefore, the radius of circle is 10 cm.
APPEARS IN
संबंधित प्रश्न
Write True or False. Give reason for your answer.
Sector is the region between the chord and its corresponding arc.
true or false
A circle is a plane figure.
In the below fig. O is the centre of the circle. If ∠APB = 50°, find ∠AOB and ∠OAB.

In the given figure, a circle inscribed in a triangle ABC, touches the sides AB, BC and AC at points D, E and F Respectively. If AB= 12cm, BC=8cm and AC = 10cm, find the length of AD, BE and CF.
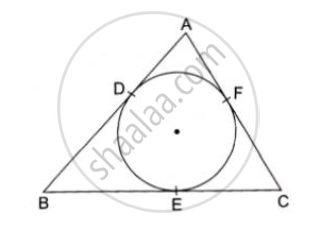
In Fig. 4, a circle inscribed in triangle ABC touches its sides AB, BC and AC at points D, E and F respectively. If AB = 12 cm, BC = 8 cm and AC = 10 cm, then find the lengths of AD, BE and CF.
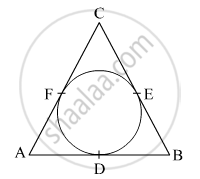
In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

The circle which passes through all the vertices of a triangle is called ______.
Suppose you are given a circle. Describe a method by which you can find the center of this circle.
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)

If a number of circles touch a given line segment PQ at a point A, then their centres lie on the perpendicular bisector of PQ.
