Advertisements
Advertisements
प्रश्न
If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
उत्तर

Consider a ΔABC.
Two circles are drawn while taking AB and AC as the diameter.
Let they intersect each other at D and let D not lie on BC.
Join AD.
∠ADB = 90° ...(Angle subtended by semi-circle)
∠ADC = 90° ...(Angle subtended by semi-circle)
∠BDC = ∠ADB + ∠ADC = 90° + 90° = 180°
Therefore, BDC is a straight line and hence, our assumption was wrong.
Thus, Point D lies on third side BC of ΔABC.
APPEARS IN
संबंधित प्रश्न
If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
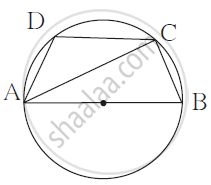
In the figure, `square`ABCD is a cyclic quadrilateral. Seg AB is a diameter. If ∠ ADC = 120˚, complete the following activity to find measure of ∠ BAC.
`square` ABCD is a cyclic quadrilateral.
∴ ∠ ADC + ∠ ABC = 180°
∴ 120˚ + ∠ ABC = 180°
∴ ∠ ABC = ______
But ∠ ACB = ______ .......(angle in semicircle)
In Δ ABC,
∠ BAC + ∠ ACB + ∠ ABC = 180°
∴ ∠BAC + ______ = 180°
∴ ∠ BAC = ______
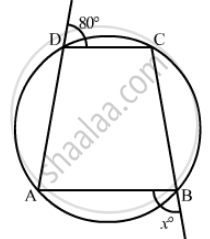
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

In the given figure, ABCD is a cyclic quadrilateral. Find the value of x.
Find all the angles of the given cyclic quadrilateral ABCD in the figure.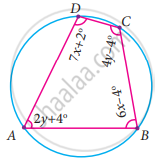
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to ______.
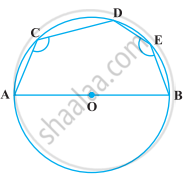
In the following figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD + ∠BED.
ABCD is a parallelogram. A circle through A, B is so drawn that it intersects AD at P and BC at Q. Prove that P, Q, C and D are concyclic.
If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
