Advertisements
Advertisements
рдкреНрд░рд╢реНрди
if `cot theta = 3/4` prove that `sqrt((sec theta - cosec theta)/(sec theta +cosec theta)) = 1/sqrt7`
рдЙрддреНрддрд░
`cot theta = "ЁЭСОЁЭССЁЭСЧЁЭСОЁЭСРЁЭСТЁЭСЫЁЭСб ЁЭСаЁЭСЦЁЭССЁЭСТ"/"ЁЭСЬЁЭСЭЁЭСЭЁЭСЬЁЭСаЁЭСЦЁЭСбЁЭСТ ЁЭСаЁЭСЦЁЭССЁЭСТ"`
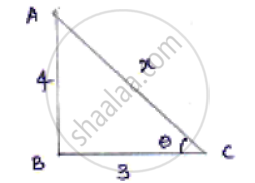
Let x be the hypotenuse by applying Pythagoras theorem.
ЁЭР┤ЁЭР╢2 = ЁЭР┤ЁЭР╡2 + ЁЭР╡ЁЭР╢2
ЁЭСе2 = 16 + 9
`x^2 = 25 => x = 5`
`sec theta = (AC)/(AB) = 5/4`
`cosec theta = (AC)/(AB) = 5/4`
On substituting in equation we get
`sqrt((sec theta - cosec theta)/(sec theta + cosec theta)) = sqrt((5/3 - 5/4)/(5/3 + 5/4))`
`= sqrt(((20 - 15)/12)/((20 + 15)/12)) = sqrt(5/35) = 1/sqrt7`
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНтАНрди
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin A = 2/3`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`cos A = 4/5`
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sec theta = 13/5`
If `tan theta = a/b`, find the value of `(cos theta + sin theta)/(cos theta - sin theta)`
Evaluate the following
cos 60° cos 45° - sin 60° тИЩ sin 45°
Evaluate the following
cos2 30° + cos2 45° + cos2 60° + cos2 90°
Evaluate the Following
4(sin4 30° + cos2 60°) − 3(cos2 45° − sin2 90°) − sin2 60°
Prove that: cot θ + tan θ = cosec θ·sec θ
Proof: L.H.S. = cot θ + tan θ
= `square/square + square/square` ......`[тИ╡ cot θ = square/square, tan θ = square/square]`
= `(square + square)/(square xx square)` .....`[тИ╡ square + square = 1]`
= `1/(square xx square)`
= `1/square xx 1/square`
= cosec θ·sec θ ......`[тИ╡ "cosec" θ = 1/square, sec θ = 1/square]`
= R.H.S.
∴ L.H.S. = R.H.S.
∴ cot θ + tan θ = cosec·sec θ
If cosec θ = `("p" + "q")/("p" - "q")` (p ≠ q ≠ 0), then `|cot(π/4 + θ/2)|` is equal to ______.
If θ is an acute angle and sin θ = cos θ, find the value of tan2 θ + cot2 θ – 2.
