Advertisements
Advertisements
प्रश्न
If |x + 2| ≤ 9, then ______.
पर्याय
x ∈ (–7, 11)
x ∈ [–11, 7]
x ∈ (–∞, –7) ∪ (11, ∞)
x ∈ (–∞, –7) ∪ [11, ∞)
उत्तर
If |x + 2| ≤ 9, then x ∈ [– 11, 7].
Explanation:
Given that |x + 2| ≤ 9
⇒ –9 ≤ x + 2 ≤ 9
⇒ –9 – 2 ≤ x ≤ 9 – 2 ......[|x| < a ⇒ –a ≤ x ≤ a]
⇒ –11 ≤ x ≤ 7
⇒ x ∈ [–11, 7]
APPEARS IN
संबंधित प्रश्न
Solve: −4x > 30, when x ∈ R
x + 5 > 4x − 10
3x + 9 ≥ −x + 19
−(x − 3) + 4 < 5 − 2x
\[\frac{5x}{2} + \frac{3x}{4} \geq \frac{39}{4}\]
\[\frac{2x + 3}{4} - 3 < \frac{x - 4}{3} - 2\]
\[\frac{2x + 3}{5} - 2 < \frac{3\left( x - 2 \right)}{5}\]
\[x - 2 \leq \frac{5x + 8}{3}\]
\[\frac{6x - 5}{4x + 1} < 0\]
\[\frac{2x - 3}{3x - 7} > 0\]
\[\frac{7x - 5}{8x + 3} > 4\]
Solve each of the following system of equations in R.
3x − 6 > 0, 2x − 5 > 0
Solve the following system of equation in R.
x + 5 > 2(x + 1), 2 − x < 3 (x + 2)
Solve each of the following system of equations in R.
\[\frac{7x - 1}{2} < - 3, \frac{3x + 8}{5} + 11 < 0\]
Solve \[\frac{\left| x - 2 \right|}{x - 2} > 0\]
Solve \[\left| x - 1 \right| + \left| x - 2 \right| + \left| x - 3 \right| \geq 6\]
Mark the correct alternative in each of the following:
The linear inequality representing the solution set given in 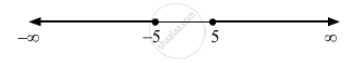
Solve the inequality, 3x – 5 < x + 7, when x is a whole number.
Solve the inequality, 3x – 5 < x + 7, when x is an integer.
Solve the following system of inequalities:
`x/(2x + 1) ≥ 1/4, (6x)/(4x - 1) < 1/2`
If x ≥ –3, then x + 5 ______ 2.
If –x ≤ –4, then 2x ______ 8.
If `1/(x - 2) < 0`, then x ______ 2.
If |3x – 7| > 2, then x ______ `5/3` or x ______ 3.
The longest side of a triangle is twice the shortest side and the third side is 2cm longer than the shortest side. If the perimeter of the triangle is more than 166 cm then find the minimum length of the shortest side.
x and b are real numbers. If b > 0 and |x| > b, then ______.
If |x − 1| > 5, then ______.
If `(-3)/4 x ≤ – 3`, then x ______ 4.
