Advertisements
Advertisements
प्रश्न
if z = x + iy, `w = (2 -iz)/(2z - i)` and |w| = 1. Find the locus of z and illustrate it in the Argand Plane.
उत्तर
z =x + iy
`w = (2 - iz)/(2z - i)`
`= (2 - i(x + iy))/(2(x + iy) - i)`
`= (2 - ix - i^2y)/(2x + 2iy - i)`
`w = ((2 + y) - ix)/(2x +(2y - 1)i)`
`|w| = |((2+y) - ix)/(2x + (2y - 1)i)|`
`1 = sqrt((2+y)^2 + x^2)/sqrt((2x)^2 + (2y - 1)^2)`
`4x^2 + 4y^2 - 4y + 1 = 4 +4y + y^2 + x^2`
`3x^2 + 3y^2 - 8y - 3 = 0`
`x^2 + y^2 - 8/3 y - 1 = 0`
∴ Locus is a circle
with centre `(0, 4/3)`
Radius = `sqrt(16/9 + 1) = 5/3`
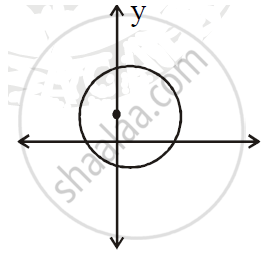
APPEARS IN
संबंधित प्रश्न
Write the sum of intercepts cut off by the plane `vecr.(2hati+hatj-k)-5=0` on the three axes
Find the intercepts cut off by the plane 2x + y – z = 5.
Prove that if a plane has the intercepts a, b, c and is at a distance of P units from the origin, then `1/a^2 + 1/b^2 + 1/c^2 = 1/p^2`
A variable plane which remains at a constant distance 3p from the origin cuts the coordinate axes at A, B, C. Show that the locus of the centroid of triangle ABC is `1/x^2 + 1/y^2 + 1/z^2 = 1/p^2`
Write the equation of the plane whose intercepts on the coordinate axes are 2, −3 and 4.
Reduce the equations of the following planes to intercept form and find the intercepts on the coordinate axes.
2x − y + z = 5
A plane meets the coordinate axes at A, B and C, respectively, such that the centroid of triangle ABC is (1, −2, 3). Find the equation of the plane.
Find the equation of the plane with intercept 3 on the y-axis and parallel to the ZOX plane.
Find the equation of the plane through the point \[2 \hat{i} + \hat{j} - \hat{k} \] and passing through the line of intersection of the planes \[\vec{r} \cdot \left( \hat{i} + 3 \hat{j} - \hat{k} \right) = 0 \text{ and } \vec{r} \cdot \left( \hat{j} + 2 \hat{k} \right) = 0 .\]
Find the equation of the plane passing through the line of intersection of the planes 2x − y = 0 and 3z − y = 0 and perpendicular to the plane 4x + 5y − 3z = 8
Find the equation of the plane through the line of intersection of the planes x + 2y + 3z + 4 = 0 and x − y + z + 3 = 0 and passing through the origin.
Find the vector equation (in scalar product form) of the plane containing the line of intersection of the planes x − 3y + 2z − 5 = 0 and 2x − y + 3z − 1 = 0 and passing through (1, −2, 3).
Find the equation of the plane that is perpendicular to the plane 5x + 3y + 6z + 8 = 0 and which contains the line of intersection of the planes x + 2y + 3z − 4 = 0, 2x + y − z + 5 = 0.
Find the equation of the plane through the line of intersection of the planes \[\vec{r} \cdot \left( \hat{i} + 3 \hat{j} \right) + 6 = 0 \text{ and } \vec{r} \cdot \left( 3 \hat{i} - \hat{j} - 4 \hat{k} \right) = 0,\] which is at a unit distance from the origin.
Find the equation of the plane passing through the intersection of the planes \[\vec{r} \cdot \left( 2 \hat{i} + \hat{j} + 3 \hat{k} \right) = 7, \vec{r} \cdot \left( 2 \hat{i} + 5 \hat{j} + 3 \hat{k} \right) = 9\] and the point (2, 1, 3).
Find the vector equation of the plane through the line of intersection of the planes x + y+ z = 1 and 2x + 3y + 4z = 5 which is perpendicular to the plane x − y + z = 0.
Find the vector equation of the plane passing through the intersection of the planes
\[\vec{r} \cdot \left( \hat{ i } + \hat{ j }+ \hat{ k }\right) = \text{ 6 and }\vec{r} \cdot \left( \text{ 2 } \hat{ i} +\text{ 3 } \hat{ j } + \text{ 4 } \hat{ k } \right) = - 5\] and the point (1, 1, 1).
Find the equation of the plane through the line of intersection of the planes \[x + y + z =\]1 and 2x \[+\] 3 \[+\] y \[+\] 4\[z =\] 5 and twice of its \[y\] -intercept is equal to three times its \[z\]-intercept
Find the length of the perpendicular from origin to the plane `vecr. (3i - 4j-12hatk)+39 = 0`
Find the locus of a complex number, z = x + iy, satisfying the relation `|[ z -3i}/{z +3i]| ≤ sqrt2 `. Illustrate the locus of z in the Argand plane.
The intercepts made by the plane 2x – 3y + 5z + 4 = 0 on the coordinate axes are `-2, 4/3, (-4)/5`.
The intercepts made on the coordinate axes by the plane 2x + y − 2z = 3 are:
