Advertisements
Advertisements
प्रश्न
In a right-angled triangle ABC. ∠ABC = 90° and D is the midpoint of AC. Prove that BD = `(1)/(2)"AC"`.
उत्तर
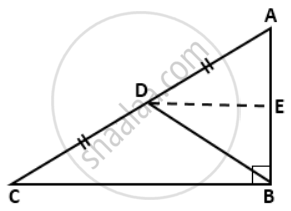
Draw line segment DE || CB, which meets AB at point E.
Now, DE || CB and AB is the transversal,
∴ ∠AED = ∠ABC ....(corrresponding angles)
∠ABC = 90° ....(given)
⇒ ∠AED = 90°
Also, as D is the mid-point of AC and DE || CB,
DE bisects side AB,
I.e. AE = BE ....(i)
In ΔAED and ΔBED,
∠AED = ∠BED ....(Each 90°)
AE = BE ....[From (i)]
DE = DE ....(Common)
∴ ΔAED ≅ ΔBEd ....(By SAS Test)
⇒ AD = BD ....(C.P.C.T.C)
⇒ BD = AC
⇒ BD = `(1)/(2)"AC"`.
APPEARS IN
संबंधित प्रश्न
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is rectangle.
L and M are the mid-point of sides AB and DC respectively of parallelogram ABCD. Prove that segments DL and BM trisect diagonal AC.
ABCD is a quadrilateral in which AD = BC. E, F, G and H are the mid-points of AB, BD, CD and Ac respectively. Prove that EFGH is a rhombus.

ABCD is a kite in which BC = CD, AB = AD. E, F and G are the mid-points of CD, BC and AB respectively. Prove that: The line drawn through G and parallel to FE and bisects DA.
In the given figure, PS = 3RS. M is the midpoint of QR. If TR || MN || QP, then prove that: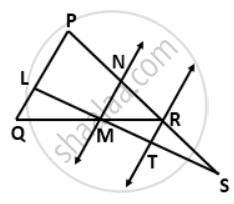
ST = `(1)/(3)"LS"`
In the given figure, T is the midpoint of QR. Side PR of ΔPQR is extended to S such that R divides PS in the ratio 2:1. TV and WR are drawn parallel to PQ. Prove that T divides SU in the ratio 2:1 and WR = `(1)/(4)"PQ"`.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if ______.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is ______.
