Advertisements
Advertisements
प्रश्न
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
उत्तर
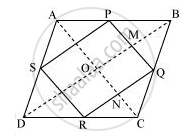
In ΔABC, P and Q are the mid-points of sides AB and BC respectively.
∴ PQ || AC and PQ = `1/2 AC` ...(Using mid-point theorem) ...(1)
In ΔADC,
R and S are the mid-points of CD and AD respectively.
∴ RS || AC and RS = `1/2 AC` ...(Using mid-point theorem) ...(2)
From equations (1) and (2), we obtain
PQ || RS and PQ = RS
Since in quadrilateral PQRS, one pair of opposite sides is equal and parallel to each other, it is a parallelogram.
Let the diagonals of rhombus ABCD intersect each other at point O.
In quadrilateral OMQN,
MQ || ON ...(∵ PQ || AC)
QN || OM ...(∵ QR || BD)
Therefore, OMQN is a parallelogram.
⇒ ∠MQN = ∠NOM
⇒ ∠PQR = ∠NOM
However, ∠NOM = 90° ...(Diagonals of a rhombus are perpendicular to each other)
∴ ∠PQR = 90°
Clearly, PQRS is a parallelogram having one of its interior angles as 90°.
Hence, PQRS is a rectangle.
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
- D is the mid-point of AC
- MD ⊥ AC
- CM = MA = `1/2AB`
ABCD is a kite having AB = AD and BC = CD. Prove that the figure formed by joining the
mid-points of the sides, in order, is a rectangle.
Fill in the blank to make the following statement correct:
The figure formed by joining the mid-points of consecutive sides of a quadrilateral is
In parallelogram ABCD, P is the mid-point of DC. Q is a point on AC such that CQ = `(1)/(4)"AC"`. PQ produced meets BC at R. Prove that
(i) R is the mid-point of BC, and
(ii) PR = `(1)/(2)"DB"`.
In AABC, D and E are two points on the side AB such that AD = DE = EB. Through D and E, lines are drawn parallel to BC which meet the side AC at points F and G respectively. Through F and G, lines are drawn parallel to AB which meet the side BC at points M and N respectively. Prove that BM = MN = NC.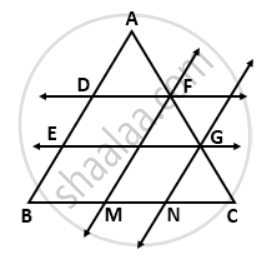
In the given figure, PS = 3RS. M is the midpoint of QR. If TR || MN || QP, then prove that: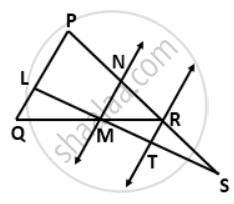
ST = `(1)/(3)"LS"`
E is the mid-point of a median AD of ∆ABC and BE is produced to meet AC at F. Show that AF = `1/3` AC.
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.
D, E and F are respectively the mid-points of the sides AB, BC and CA of a triangle ABC. Prove that by joining these mid-points D, E and F, the triangles ABC is divided into four congruent triangles.
