Advertisements
Advertisements
प्रश्न
D, E and F are respectively the mid-points of the sides AB, BC and CA of a triangle ABC. Prove that by joining these mid-points D, E and F, the triangles ABC is divided into four congruent triangles.
उत्तर
Given: In a ΔABC, D, E and F are respectively the mid-points of the sides AB, BC and CA.
To prove: ΔABC is divided into four congruent triangles.
Proof: Since, ABC is a triangle and D, E and F are the mid-points of sides AB, BC and CA, respectively.
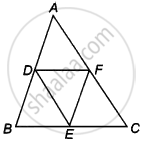
Then, AD = BD = `1/2`AB, BE = EC = `1/2`BC
And AF = CF = `1/2`AC
Now, using the mid-point theorem,
EF || AB and EF = `1/2`AB = AD = BD
ED || AC and ED = `1/2`AC = AF = CF
And DF || BC and DF = `1/2`BC = BE = CE
In ΔADF and ΔEFD,
AD = EF
AF = DE
And DF = FD ...[Common]
∴ ΔADF ≅ ΔEFD ...[By SSS congruence rule]
Similarly, ΔDEF ≅ ΔEDB
And ΔDEF ≅ ΔCFE
So, ΔABC is divided into four congruent triangles.
Hence proved.
APPEARS IN
संबंधित प्रश्न
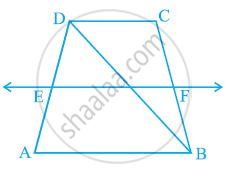
ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see the given figure). Show that F is the mid-point of BC.
ABCD is a rhombus, EABF is a straight line such that EA = AB = BF. Prove that ED and FC when produced meet at right angles
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
The following figure shows a trapezium ABCD in which AB // DC. P is the mid-point of AD and PR // AB. Prove that:
PR = `[1]/[2]` ( AB + CD)

In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P.
Prove that:
(i) BP = 2AD
(ii) O is the mid-point of AP.
In ΔABC, BE and CF are medians. P is a point on BE produced such that BE = EP and Q is a point on CF produced such that CF = FQ. Prove that: A is the mid-point of PQ.
Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
AD is a median of side BC of ABC. E is the midpoint of AD. BE is joined and produced to meet AC at F. Prove that AF: AC = 1 : 3.
In ΔABC, the medians BE and CD are produced to the points P and Q respectively such that BE = EP and CD = DQ. Prove that: Q A and P are collinear.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
