Advertisements
Advertisements
प्रश्न
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.
उत्तर
Given: In a square ABCD, P, Q, R and S are the mid-points of AB, BC, CD and DA, respectively.
To show: PQRS is a square.
Construction: Join AC and BD.

Proof: Since, ABCD is a square.
∴ AB = BC = CD = AD
Also, P, Q, R and S are the mid-points of AB, BC, CD and DA, respectively.
Then, in ΔADC, SR || AC
And SR = `1/2`AC [By mid-point theorem] ...(i)
In ΔABC, PQ || AC
And PQ = `1/2`AC ...(ii)
From equations (i) and (ii),
SR || PQ and SR = PQ = `1/2`AC ...(iii)
Similarly, SP || BD and BD || RQ
∴ SP || RQ and SP = `1/2`BD
And RQ = `1/2`BD
∴ SP = RQ = `1/2`BD
Since, diagonals of a square bisect each other at right angle.
∴ AC = BD
⇒ SP = RQ = `1/2`AC ...(iv)
From equations (iii) and (iv),
SR = PQ = SP = RQ ...[All side are equal]
Now, in quadrilateral OERF,
OE || FR and OF || ER
∴ ∠EOF = ∠ERF = 90°
Hence, PQRS is a square.
Hence proved.
APPEARS IN
संबंधित प्रश्न
Fill in the blank to make the following statement correct
The triangle formed by joining the mid-points of the sides of an isosceles triangle is
In ∆ABC, E is the mid-point of the median AD, and BE produced meets side AC at point Q.
Show that BE: EQ = 3: 1.
In the given figure, AD and CE are medians and DF // CE.
Prove that: FB = `1/4` AB.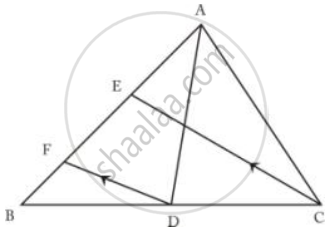
Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
If L and M are the mid-points of AB, and DC respectively of parallelogram ABCD. Prove that segment DL and BM trisect diagonal AC.
In a right-angled triangle ABC. ∠ABC = 90° and D is the midpoint of AC. Prove that BD = `(1)/(2)"AC"`.
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square is also a square.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
D, E and F are the mid-points of the sides BC, CA and AB, respectively of an equilateral triangle ABC. Show that ∆DEF is also an equilateral triangle.
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
