Advertisements
Advertisements
प्रश्न
In ∆ABC, E is the mid-point of the median AD, and BE produced meets side AC at point Q.
Show that BE: EQ = 3: 1.
उत्तर
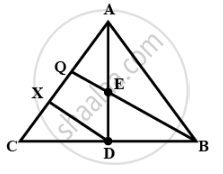
Construction: Draw DX || BQ
In ΔBCQ and ΔDCX,
∠BCQ = ∠DCX ...(Common)
∠BQC = ∠DXC ...(Corresponding angles)
So, ΔBCQ ∼ ΔDCX ....(AA Similarity criterion)
⇒ `"BQ"/"DX" = "BC"/"DC" = "CQ"/"CX"` ...(Corresponding sides are proportional.)
⇒ `"BQ"/"DX" = "2CD"/"CD"` ...(D is the mid-point of BC)
⇒ `"BQ"/"DX" = 2` ...(i)
Similarly, ΔAEQ ∼ ΔADX,
⇒ `"EQ"/"DX" = "AE"/"ED" = 1/2` ...(E is the mid-point of AD)
That is `"EQ"/"DX" = 1/2` ...(ii)
Dividing (i) by (ii), We get
⇒ `"BQ"/"EQ" = 4`
⇒ BE + EQ = 4EQ
⇒ BE = 3EQ
⇒ `"BQ"/"EQ" = 3/1`
APPEARS IN
संबंधित प्रश्न
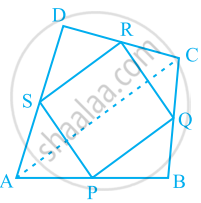
ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see the given figure). AC is a diagonal. Show that:
- SR || AC and SR = `1/2AC`
- PQ = SR
- PQRS is a parallelogram.
ABC is a triang D is a point on AB such that AD = `1/4` AB and E is a point on AC such that AE = `1/4` AC. Prove that DE = `1/4` BC.
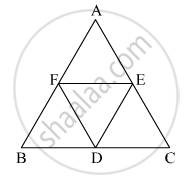
In the given figure, ΔABC is an equilateral traingle. Points F, D and E are midpoints of side AB, side BC, side AC respectively. Show that ΔFED is an equilateral traingle.
In the given figure, M is mid-point of AB and DE, whereas N is mid-point of BC and DF.
Show that: EF = AC.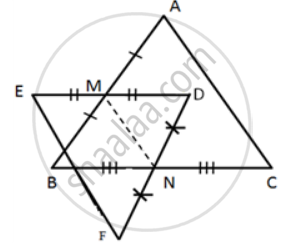
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find FE, if BC = 14 cm
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: AB, if DC = 8 cm and PQ = 9.5 cm
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 4CR = AB.
In ∆ABC, AB = 5 cm, BC = 8 cm and CA = 7 cm. If D and E are respectively the mid-points of AB and BC, determine the length of DE.
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.
D, E and F are respectively the mid-points of the sides AB, BC and CA of a triangle ABC. Prove that by joining these mid-points D, E and F, the triangles ABC is divided into four congruent triangles.
