Advertisements
Advertisements
प्रश्न
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square is also a square.
उत्तर

Join AC and BD
In ΔACD, G and H are the mid-points of DC and AC respectively.
Therefore, GH || AC and GH = `(1)/(2)"AC"` ......(i)
In ΔABC, E and F are the mid-points of AB and BC respectively.
Therefore, EF || AC and EF = `(1)/(2)"AC"` ......(i)
From (i) and (ii)
EF || GH and EF = GH = `(1)/(2)"AC"` .........(iii)
Similarly, it can be proved that
EF || GH and EH = GF = `(1)/(2)"BD"` .........(iv)
But AC = BD ...(diagonals of a square are equal)
Dividing both sides by 2,
`(1)/(2)"BD" = (1)/(2)"AC"` (iv)
From (iii) and (iv)
EF = Gh = EH = GF
Therefore, EFGH is a parallelogram.
Now in ΔGOH and ΔGOF
OH = OF ...(diagonals of a parallelogram bisect each other)
OG = O ...(common)
GH = GF
∴ ΔGOH ≅ ΔGOF
∴ ∠GOH = ∠GOF
Now,
∠GOH +∠GOF = 180°
⇒ ∠GOH + ∠GOH = 180°
⇒ 2∠GOH = 180°
⇒ ∠GOH = 90°
Therefore, diagonals of parallelogram EFGH bisect each other and are perpendicular to each other.
Thus, EFGH is a square.
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see the given figure). AC is a diagonal. Show that:
- SR || AC and SR = `1/2AC`
- PQ = SR
- PQRS is a parallelogram.
In a triangle ∠ABC, ∠A = 50°, ∠B = 60° and ∠C = 70°. Find the measures of the angles of
the triangle formed by joining the mid-points of the sides of this triangle.
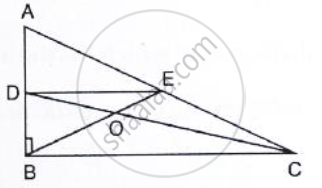
In Fig. below, triangle ABC is right-angled at B. Given that AB = 9 cm, AC = 15 cm and D,
E are the mid-points of the sides AB and AC respectively, calculate
(i) The length of BC (ii) The area of ΔADE.
In below Fig, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

In the given figure, `square`PQRS and `square`MNRL are rectangles. If point M is the midpoint of side PR then prove that,
- SL = LR
- LN = `1/2`SQ

In triangle ABC, the medians BP and CQ are produced up to points M and N respectively such that BP = PM and CQ = QN. Prove that:
- M, A, and N are collinear.
- A is the mid-point of MN.
In the given figure, AD and CE are medians and DF // CE.
Prove that: FB = `1/4` AB.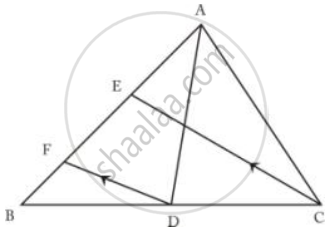
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: AB, if DC = 8 cm and PQ = 9.5 cm
ABCD is a kite in which BC = CD, AB = AD. E, F and G are the mid-points of CD, BC and AB respectively. Prove that: ∠EFG = 90°
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
