Advertisements
Advertisements
प्रश्न
In a ΔABC, if ∠A = 60°, ∠B = 80° and the bisectors of ∠B and ∠C meet at O, then ∠BOC =
पर्याय
60°
120°
150°
30°
उत्तर
In the given ΔABC,∠A = 60° and ∠B = 80° . Bisectors of ∠B and ∠C meet at O.
We need to find ∠BOC
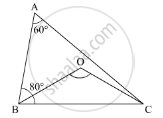
Since, OB is the bisector of ∠B.
Thus, `∠OBC = 1/2 ∠ABC ..... (1)`
Now, using the angle sum property of the triangle
In ΔABC, we get,
∠A + ∠B + ∠C =180°
60° + 80° + ∠C = 180°
140° + ∠C = 180°
∠C = 180° - 140°
∠C = 40°
Similarly, in ΔBOC
∠OBC + ∠O + ∠OCB = 180
∠O + 20° + 40°=180°
∠O + 60° = 180°
∠O = 180° - 60°
= 120°
Hence, ∠BOC = 120°
APPEARS IN
संबंधित प्रश्न
The vertical angle of an isosceles triangle is 100°. Find its base angles.
Find the measure of each exterior angle of an equilateral triangle.
ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that ΔABC is isosceles
Which of the following statements are true (T) and which are false (F) :
If the altitude from one vertex of a triangle bisects the opposite side, then the triangle may be isosceles.
Which of the following statements are true (T) and which are false (F):
The two altitudes corresponding to two equal sides of a triangle need not be equal.
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
Fill in the blank to make the following statement true.
The sum of any two sides of a triangle is .... than the third side.
D is a point on the side BC of a ∆ABC such that AD bisects ∠BAC. Then ______.
Two sides of a triangle are of lengths 5 cm and 1.5 cm. The length of the third side of the triangle cannot be ______.
In ∆PQR, ∠P = 70° and ∠R = 30°. Which side of this triangle is the longest? Give reason for your answer.
