Advertisements
Advertisements
प्रश्न
In a circle of radius 10.5 cm, the minor arc is one-fifth of the major arc. Find the area of the sector corresponding to the major arc.
उत्तर
Let the length of the major arc be x cm
Radius of the circle = 10.5 cm
∴ Length of the minor arc`=x/5 "cm"`
Circumference `= (x + x/5) = "6x"/5 "cm"`
Using the given data, we get :
`(6x)/5 = 2xx22/7xx21/2`
`=>(6x)/5 = 66`
Or.
x = 55
∴ Area of the sector corresponding to the major arc `= (1/2xx55xx21/2) = 288.75 "cm"^2`
APPEARS IN
संबंधित प्रश्न
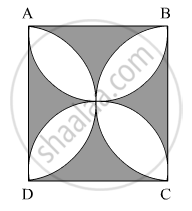
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
From a circular sheet of radius 4 cm, a circle of radius 3 cm is removed. Find the area of the remaining sheet. (Take π = 3.14)

In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find Circumference of the circle
Four equal circles each of radius a, touch each other. Show that area between them is `6/7a^2`
In the given figure, OPQR is a rhombus, three of whose vertices lie on a circle with centre O. If the area of the rhombus is `32sqrt(3)`, find the radius of the circle.
The wheels of a car make 2500 revolutions in covering a distance of 4.95 km. Find the diameter of a wheel.
The wheel of the car makes 10 revolutions per second. If its diameter is 70 cm, find the speed of the car in km per hour.
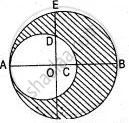
In the adjoining figure, the crescent is formed by two circles which touch at the point A. O is the centre of bigger circle. If CB = 9 cm and DE = 5 cm, find the area of the shaded portion.
The diameter of a cycle wheel is `4(5)/(11)"cm"`. How many revolutions will it make in moving 6.3km?
Find the number of revolutions made by a circular wheel of area 1.54 m2 in rolling a distance of 176 m.
