Question

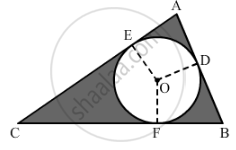
In the given figure, ∆ABC is right-angled at A. Find the area of the shaded region if AB = 6 cm, BC = 10 cm and O is the centre of the incircle of ∆ABC.

Answer 1

Using Pythagoras' theorem for triangle ABC, we have:

CA² + AB² = BC² 

CA` = sqrt("BC"^2 - "AB"^2)`

`=sqrt(100-36)`

`=sqrt(64)`

= 8 cm

Now, we must find the radius of the incircle. Draw OE, OD and OF perpendicular to AC, AB and BC, respectively.

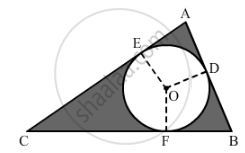
Consider quadrilateral AEOD.
Here,

EO = OD(Both are radii.)

Because the circle is an incircle, AE and AD are tangents to the circle.

∠AEO = ∠ADO = 90° 

Also,

∠A = 90° 

Therefore, AEOD is a square.

Thus, we can say that AE = EO = OD = AD = r.

CE =  CF = 8 - r

BF = BD = 6 - r

CF + BF = 10

⇒ (8 - r)+(6-r) = 10

⇒ 14 - 2r = 10

⇒ r = 2 cm

Area of the shaded part = Area of the triangle-- Area of the circle

`= {1/2xx6xx8} - {pixx2xx2}`

= 24 - 12.56 

= 11.44 cm²