Advertisements
Advertisements
प्रश्न
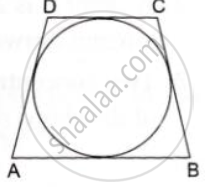
In the given figure, a circle touches all the four sides of a quadrilateral ABCD whose three sides are AB = 6cm, BC=7cm and CD=4 cm. Find AD.
उत्तर

Let the circle touch the sides of the quadrilateral AB, BC, CD and DA at P, Q, R and S respectively.
Given, AB = 6cm, BC = 7 cm and CD = 4cm.
Tangents drawn from an external point are equal.
∴ AP = AS, BP = BQ,CR = CQ and DR = DS
Now, AB + CD (AP + BP) + (CR + DR)
⇒AB + CD= (AS+ BQ) + (CQ+ DS)
⇒ AB +CD +(AS+ DS) +(BQ+ CQ)
⇒ AB +CD =AD+ BC
⇒AD= ( AB+ CD)- BC
⇒ AD=(6+4)-7
⇒ AD = 3 cm.
∴ The length of AD is 3 cm.
APPEARS IN
संबंधित प्रश्न
Find the length of the tangent drawn from a point whose distance from the centre of a circle is 25 cm. Given that the radius of the circle is 7 cm.
In the given figure, the chord AB of the larger of the two concentric circles, with center O, touches the smaller circle at C. Prove that AC = CB.

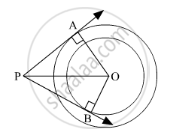
In fig. 3 are two concentric circles of radii 6 cm and 4 cm with centre O. If AP is a tangent to the larger circle and BP to the smaller circle and length of AP is 8 cm, find the length of BP ?
The circumference of a circle is 22 cm. The area of its quadrant (in cm2) is
If the length of a chord of a circle is 16 cm and is at a distance of 15 cm from the centre of the circle, then the radius of the circle is
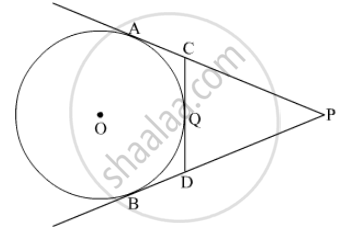
In the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA = 12 cm, QC = QD = 3 cm, then find PC + PD.
If all the sides of a parallelogram touch a circle, show that the parallelogram is a rhombus.
ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
Find the diameter of the circle
Radius = 10 cm
The length of tangent from an external point on a circle is always greater than the radius of the circle.
