Advertisements
Advertisements
प्रश्न
In the given figure, if ∠BAC = 60° and ∠BCA = 20°, find ∠ADC.

उत्तर
It is given that, `angle BAC = 60°` and `angle BCA = 20°`
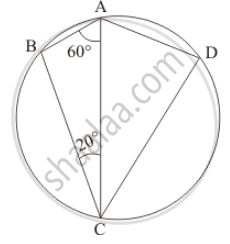
We have to find the `angle ADC `
In given Δ ABC we have
`angle ABC + angle BCA + angle BAC `= 180° \[ \left( \text{ Angle sum property } \right)\]
\[ \Rightarrow \angle ABC = 180° - \left( 60° + 20° \right) = 100° \]
In cyclic quadrilateral ABCD we have
`angle B + angleD = 180°` (Sum of pair of opposite angles of a cyclic quadilateral is 180º)
Then,
`angle D = 180° - 100° `
`angle D = 80° `
Hence `angle ADC = 80°`
APPEARS IN
संबंधित प्रश्न
Fill in the blanks:
The centre of a circle lies in ____________ of the circle.
Write True or False. Give reasons for your answers.
If a circle is divided into three equal arcs, each is a major arc.
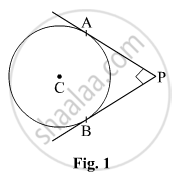
In Fig. 1, PA and PB are two tangents drawn from an external point P to a circle with centre C and radius 4 cm. If PA ⊥ PB, then the length of each tangent is:
In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

The circle which passes through all the vertices of a triangle is called ______.
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)

If the radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other circle is ______
In the adjoining figure, Δ ABC is circumscribing a circle. Then, the length of BC is ______

In the given figure, AB is the diameter of the circle. Find the value of ∠ACD.

If AOB is a diameter of a circle and C is a point on the circle, then AC2 + BC2 = AB2.
