Advertisements
Advertisements
प्रश्न
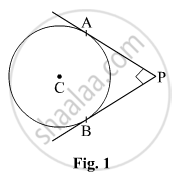
In Fig. 1, PA and PB are two tangents drawn from an external point P to a circle with centre C and radius 4 cm. If PA ⊥ PB, then the length of each tangent is:
पर्याय
(A) 3 cm
(B) 4 cm
(C) 5 cm
(D) 6 cm
उत्तर
Since `APbotPB,CA botAP,CBbotBP `
and AC = CB = radius of the circle, therefore APBC is a square having side equal to 4 cm.
Therefore, length of each tangent is 4 cm.
APPEARS IN
संबंधित प्रश्न
In Figure 1, common tangents AB and CD to the two circles with centres 01and 02 intersect at E. Prove that AB = CD.

If the quadrilateral sides touch the circle prove that sum of pair of opposite sides is equal to the sum of other pair.
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

If all the sides of a parallelogram touch a circle, show that the parallelogram is a rhombus.
In the following figure, tangents PQ and PR are drawn to a circle such that ∠RPQ = 30°. A chord RS is drawn parallel to the tangent PQ, then ∠RQS.

Let s denote the semi-perimeter of a triangle ABC in which BC = a, CA = b, AB = c. If a circle touches the sides BC, CA, AB at D, E, F, respectively, prove that BD = s – b.
In figure, tangents PQ and PR are drawn to a circle such that ∠RPQ = 30°. A chord RS is drawn parallel to the tangent PQ. Find the ∠RQS.
[Hint: Draw a line through Q and perpendicular to QP.]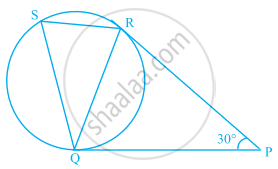
In the following figure, ∠ADC = 130° and chord BC = chord BE. Find ∠CBE.

In the given figure, O is the centre of the circle. If ∠ AOB = 145°, then find the value of x.

