Advertisements
Advertisements
प्रश्न
If the quadrilateral sides touch the circle prove that sum of pair of opposite sides is equal to the sum of other pair.
उत्तर
Consider a quadrilateral ABCD touching circle with center O at points E, F, G and H as in figure.

We know that
The tangents drawn from same external points to the circle are equal in length.
1. Consider tangents from point A [AM ⊥ AE]
AH = AE …. (i)
2. From point B [EB & BF]
BF = EB …. (ii)
3. From point C [CF & GC]
FC = CG …. (iii)
4. From point D [DG & DH]
DH = DG …. (iv)
Adding (i), (ii), (iii), & (iv)
(AH + BF + FC + DH) = [(AC + CB) + (CG + DG)]
⇒ (AH + DH) + (BF + FC) = (AE + EB) + (CG + DG)
⇒ AD + BC = AB + DC [from fig.]
Sum of one pair of opposite sides is equal to other.
APPEARS IN
संबंधित प्रश्न
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
Two circles touch internally. The sum of their areas is 116 π cm2 and the distance between their centres is 6 cm. Find the radii of the circles ?
In Fig. 4, a circle inscribed in triangle ABC touches its sides AB, BC and AC at points D, E and F respectively. If AB = 12 cm, BC = 8 cm and AC = 10 cm, then find the lengths of AD, BE and CF.
An equilateral triangle ABC is inscribed in a circle with centre O. The measures of ∠BOCis

In the above figure, `square`XLMT is a rectangle. LM = 21 cm, XL = 10.5 cm. Diameter of the smaller semicircle is half the diameter of the larger semicircle. Find the area of non-shaded region.
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)

A chord is at a distance of 15 cm from the centre of the circle of radius 25 cm. The length of the chord is
If angle between two tangents drawn from a point P to a circle of radius a and centre O is 60°, then OP = `asqrt(3)`
If AB is a chord of a circle with centre O, AOC is a diameter and AT is the tangent at A as shown in figure. Prove that ∠BAT = ∠ACB
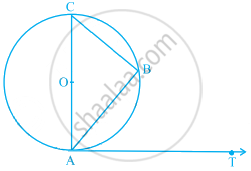
If AB = 12 cm, BC = 16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is ______.
