Advertisements
Advertisements
प्रश्न
In the given figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O' are centres of the circles. Find the area of shaded region.

उत्तर
We have,
Side of square = 28 cm and radius of each circle = \[\frac{28}{2}\] cm
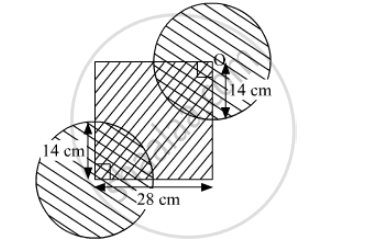
Area of the shaded region
= Area of the square + Area of the two circles − Area of the two quadrants
\[= \left( 28 \right)^2 + 2 \times \pi \times \left( \frac{28}{2} \right)^2 - 2 \times \frac{1}{4} \times \pi \times \left( \frac{28}{2} \right)^2 \]
\[ = \left( 28 \right)^2 + \frac{3}{2} \times \pi \times \left( \frac{28}{2} \right)^2 \]
\[ = \left( 28 \right)^2 \left( 1 + \frac{3}{2} \times \frac{22}{7} \times \frac{1}{2} \times \frac{1}{2} \right)\]
\[ = \left( 28 \right)^2 \left( 1 + \frac{33}{28} \right)\]
\[ = \left( 28 \right)^2 \times \frac{61}{28}\]
\[ = 28 \times 61\]
\[ = 1708 {cm}^2\]
Therefore, the area of the shaded region is 1708 cm2.
APPEARS IN
संबंधित प्रश्न
From a circular card sheet of radius 14 cm, two circles of radius 3.5 cm and a rectangle of length 3 cm and breadth 1 cm are removed. (as shown in the following figure). Find the area of the remaining sheet. (Take `pi = 22/7`)

The area of a square filed is 8 hectares. How long would a man take to cross it diagonally by walking at the rate of 4 km per hour?
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector formed by the arc. (Use π = `22/7`)
In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

Write the formula for the area of a sector of angle \[\theta\] (in degrees) of a circle of radius r.
The ratio of the outer and inner perimeters of a circular path is 23 : 22. If the path is 5 metres wide, the diameter of the inner circle is
The minute hand of a clock is 12 cm long. Find the area swept by in it 35 minutes.
A wire when bent in the form of an equilateral triangle encloses an area of `121sqrt(3)" cm"^2`. If the same wire is bent into the form of a circle, what will be the area of the circle?
A circle of the largest area is cut from a rectangular piece of cardboard with dimensions 55 cm and 42 cm. Find the ratio between the area of the circle cut and the area of the remaining card-board.
