Advertisements
Advertisements
प्रश्न
In the given figure, ∠X = 62º, ∠XYZ = 54º. If YO and ZO are the bisectors of ∠XYZ and ∠XZY respectively of ΔXYZ, find ∠OZY and ∠YOZ.

उत्तर
As the sum of all interior angles of a triangle is 180º, therefore, for ΔXYZ,
∠X + ∠XYZ + ∠XZY = 180º
62º + 54º + ∠XZY = 180º
∠XZY = 180º − 116º
∠XZY = 64º
∠OZY = 64/2 = 32º (OZ is the angle bisector of ∠XZY)
Similarly, ∠OYZ = 54/2 = 27°
Using angle sum property for ΔOYZ, we obtain
∠OYZ + ∠YOZ + ∠OZY = 180º
27º + ∠YOZ + 32º = 180º
∠YOZ = 180º − 59º
∠YOZ = 121º
APPEARS IN
संबंधित प्रश्न
In the given figure, which of the following statement is true?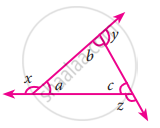
If one of the angles of a triangle is 130°, then the angle between the bisectors of the other two angles can be ______.
Two adjacent angles are equal. Is it necessary that each of these angles will be a right angle? Justify your answer.
Prove that through a given point, we can draw only one perpendicular to a given line.
[Hint: Use proof by contradiction].
In a right-angled triangle, the angles other than the right angle are ______.
If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
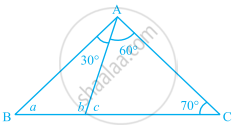
In the given figure, find the values of a, b and c.
In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
The angles of a triangle are arranged in descending order of their magnitudes. If the difference between two consecutive angles is 10°, find the three angles.
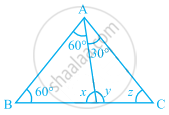
In the given figure, find the values of x, y and z.