Advertisements
Advertisements
प्रश्न
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.2911 | 0.2891 | 0.2886 | 0.2886 | 0.2885 | 0.28631 |
उत्तर
Let f(x) = `lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) |
`(sqrt(3 - 0.1) - sqrt(3))/(- 0.1)` = `(1.703 - 1.732)/(- 0.1)` = 0.29 |
`(sqrt(3 - 00.1) - sqrt(3))/(- 0.01)` = `(1.703 - 1.732)/(- 0.01)` = 0.288 |
`(sqrt(3 - 0.001) - sqrt(3))/(- 0.001)` = `(0.000284)/(- 0.001)` = 0.288 |
`(sqrt(3 + 0.001) - sqrt(3))/(- 0.001)` = `(0.000289)/(0.001)` = 0.289 |
`(sqrt(3 + 0.01) - sqrt(3))/(0.01)` = `(0.00288)/(0.01)` = 0.288 |
`(sqrt(3 + 0.1) - sqrt(3))/(0.1)` = `(0.0286)/(0.1)` = 0.286 |
`lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x` = 0.288
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit :
`lim_(x -> 1)[(x + x^2 + x^3 + ......... + x^"n" - "n")/(x - 1)]`
In the following example, given ∈ > 0, find a δ > 0 such that whenever, |x – a| < δ, we must have |f(x) – l| < ∈.
`lim_(x -> -3) (3x + 2)` = – 7
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) sin x/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.99833 | 0.99998 | 0.99999 | 0.99999 | 0.99998 | 0.99833 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 2) f(x)` where `f(x) = {{:(4 - x",", x ≠ 2),(0",", x = 2):}`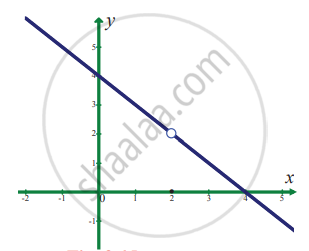
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 3) 1/(x - 3)`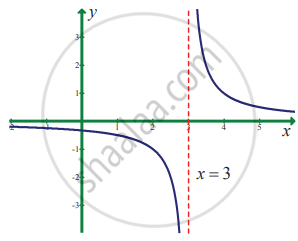
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 0) sec x`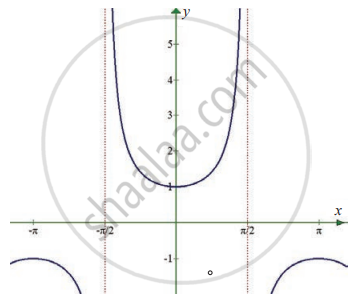
Evaluate : `lim_(x -> 3) (x^2 - 9)/(x - 3)` if it exists by finding `f(3^-)` and `f(3^+)`
Verify the existence of `lim_(x -> 1) f(x)`, where `f(x) = {{:((|x - 1|)/(x - 1)",", "for" x ≠ 1),(0",", "for" x = 1):}`
Evaluate the following limits:
`lim_(x ->) (x^"m" - 1)/(x^"n" - 1)`, m and n are integers
Evaluate the following limits:
`lim_(sqrt(x) -> 3) (x^2 - 81)/(sqrt(x) - 3)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(1 + x) - 1)/x`
Evaluate the following limits:
`lim_(x -> oo) 3/(x - 2) - (2x + 11)/(x^2 + x - 6)`
Evaluate the following limits:
`lim_(x -> oo) (x^4 - 5x)/(x^2 - 3x + 1)`
Evaluate the following limits:
`lim_(x ->oo) (x^3/(2x^2 - 1) - x^2/(2x + 1))`
Evaluate the following limits:
`lim_(x -> 0) (sinalphax)/(sinbetax)`
Evaluate the following limits:
`lim_(x-> 0) (1 - cos x)/x^2`
Choose the correct alternative:
`lim_(x -> 3) [x]` =
Choose the correct alternative:
`lim_(x -> 0) ("e"^tanx - "e"^x)/(tan x - x)` =
Choose the correct alternative:
The value of `lim_(x -> 0) sinx/sqrt(x^2)` is
If `lim_(x -> 1) (x + x^2 + x^3|+ .... + x^n - n)/(x - 1)` = 820, (n ∈ N) then the value of n is equal to ______.
