Advertisements
Advertisements
प्रश्न
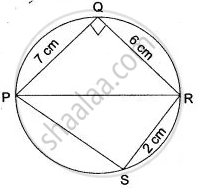
In the figure alongside PR is a diameter of the circle, PQ = 7 cm; QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.
उत्तर
PR is the diameter of the circle.
then ∠ PQR = 90° .....(Angle in semicircle)
In Δ PQR,
PR = `sqrt((7)^2 + (6)^2)`
PR = `sqrt(49 + 36)`
PR = `sqrt(85)`
Similarly,
∠ PSR = 90°
In ΔPSR,
PS = `sqrt("PR"^2 - "SR"^2)`
PS = `sqrt(85 - 4)`
PS = `sqrt(81)`
PS = 9 cm
Perimeter of cyclic quadrilateral PQRS = PQ + QR + RS + PS
Perimeter of cyclic quadrilateral PQRS = 7 cm + 6 cm + 2 cm + 9 cm = 24 cm
Perimeter of cyclic quadrilateral PQRS = 24 cm.
APPEARS IN
संबंधित प्रश्न
Prove that any four vertices of a regular pentagon are concylic (lie on the same circle).
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
Prove that the angles bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (provided they are not parallel) intersect at right triangle.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.
In following figure , Δ PQR is an isosceles teiangle with PQ = PR and m ∠ PQR = 35° .Find m ∠ QSR and ∠ QTR

ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC=120°.
Calculate: ∠ BED.
ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q. Show that quadrilateral PCDQ is cyclic.
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BAC

ABCD is a cyclic quadrilateral AB and DC are produced to meet in E. Prove that Δ EBC ∼ Δ EDA.
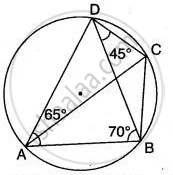
In the given below figure,
∠ BAD = 65°
∠ ABD = 70°
∠ BDC = 45°
Find: (i) ∠ BCD, (ii) ∠ ADB.
Hence show that AC is a diameter.