Advertisements
Advertisements
प्रश्न
In the given diagram, O is the centre of the circle. PR and PT are two tangents drawn from the external point P and touching the circle at Q and S respectively. MN is a diameter of the circle. Given ∠PQM = 42° and ∠PSM = 25°.
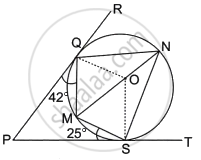
Find:
- ∠OQM
- ∠QNS
- ∠QOS
- ∠QMS
उत्तर
a. PR and PT are tangents to the circle with centre O.

Then, ∠OQP = 90°
As, radius is ⊥ to the tangent
Then, ∠OQM = ∠OQP – ∠MQP
= 90° – 42°
= 48°
b. ∠PQM = ∠QNM = 42° ...(By alternate segment theorem)
∠PSM = ∠SNM = 25°
Then ∠QNS = ∠QNM + ∠SNM
= 42° + 25°
= 67°
c. ∠QOS = 2∠QNS ...(since, angle subtended by the arc at the centre is twice the angle subtended by the arc at any other point of the circles.)
= 2 × 67°
= 134°
d. QNSN is a cyclic quadritateral
∠QNS + ∠QMS = 180°
∠QMS = 180° – 67° = 113°
APPEARS IN
संबंधित प्रश्न
If tangents PA and PB from a point P to a circle with centre O are inclined to each other an angle of 80°, then ∠POA is equal to ______.
The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
In the given figure PA = 10, PB = 2 and PC = 5. Find PD.
Two concentric circles are of radii 5 cm and 3 cm. Length of the chord of the larger circle, (in cm), which touches the smaller circle is
(A) 4
(B) 5
(C) 8
(D) 10
Prove that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
Find the angle between two radii at the centre of the circle as shown in the figure. Lines PA and PB are tangents to the circle at other ends of the radii and ∠APR = 110°.

The length of the tangent from an external point P on a circle with centre O is ______
The length of the tangent from an external point on a circle is ______
From a point P, two tangents PA and PB are drawn to a circle C(0, r). If OP = 2r, then find ∠APB. What type of triangle is APB?

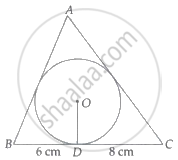
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.