Advertisements
Advertisements
प्रश्न
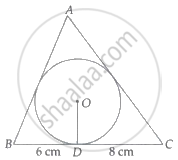
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.
उत्तर
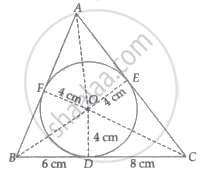
Given, BD = 6 cm, DC = 8 cm
Here, BD = BF and DC = CE .....[Tangents drawn from an external point to a circle are equal]
∴ BF = 6 cm and CE = 8 cm
Let AF = x = AE .....[Tangents drawn from external point A to the circle are equal]
In ΔABC,
a = BC = BD + DC = 6 + 8 = 14 cm
b = AC = CE + AE = (8 + x) cm
c = AB = BF + AF = (6 + x) cm
Now, s = `("a" + "b" + "c")/2`
= `(14 + (8 + "x") + (6 + "x"))/2`
= `(28 + 2"x")/2`
= (14 + x) cm
∴ Area of ΔABC = `sqrt("s"("s" - "a")("s" - "b")("s" - "c"))`
84 = `sqrt((14 + "x")(14 + "x" - 14)(14 + "x" - 8 - "x")(14 + "x" - 6 - "x"))`
84 = `sqrt("x"(14 + "x")(6)(8)`
84 = `sqrt(48"x"("x" + 14)) "cm"^2` ...(i)
`sqrt(48"x"("x" + 14))` = 84
On squaring both sides, we get
48x(x + 14) = 84 × 84
⇒ 4x(x + 14) = 84 × 7
⇒ x2 + 14x – 147 = 0
⇒ x2 + 21x – 7x – 147 = 0
x(x + 21) – 7(x + 21) = 0
(x + 21)(x – 7) = 0
So, x = 7, or x = –21 (rejected as - ve)
Hence, x = 7
Therefore, AB = c = 6 + x = 6 + 7 = 13 cm
AC = b = 8 + x = 8 + 7 = 15 cm
APPEARS IN
संबंधित प्रश्न
In the below given figure, two tangents RQ and RP are drawn from an external point R to the circle with centre O. If∠PRQ = 120°, then prove that OR = PR + RQ.

If from an external point P of a circle with centre O, two tangents PQ and PR are drawn such that ∠QPR = 120°, prove that 2PQ = PO.
Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
Find:
- ∠OBD
- ∠AOB
- ∠BED

PQ is a tangent drawn from an external point P to a circle with centre O, QOR is the diameter of the circle. If ∠POR = 120°, what is the measure of ∠OPQ?
A right circular cone is divided into three parts by trisecting its height by two planes drawn parallel to the base. Show that the volumes of the three portions starting from the top are in the ratio 1 : 7 : 19 ?
The length of tangents drawn from an external point to the circle ______
In fig, O is the centre of the circle, CA is tangent at A and CB is tangent at B drawn to the circle. If ∠ACB = 75°, then ∠AOB = ______

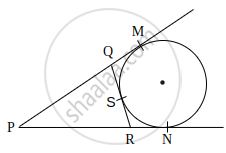
In the given figure, if a circle touches the side QR of ΔPQR at S and extended sides PQ and PR at M and N, respectively, then prove that PM = `1/2` (PQ + QR + PR)
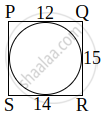
A quadrilateral PQRS is drawn to circumscribe a circle. If PQ = 12 cm, QR = 15 cm and RS = 14 cm, then find the length of SP is ______.