Advertisements
Advertisements
प्रश्न
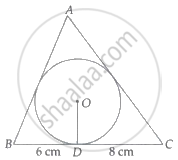
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.
उत्तर
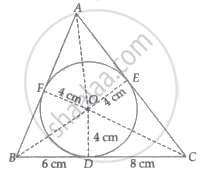
Given, BD = 6 cm, DC = 8 cm
Here, BD = BF and DC = CE .....[Tangents drawn from an external point to a circle are equal]
∴ BF = 6 cm and CE = 8 cm
Let AF = x = AE .....[Tangents drawn from external point A to the circle are equal]
In ΔABC,
a = BC = BD + DC = 6 + 8 = 14 cm
b = AC = CE + AE = (8 + x) cm
c = AB = BF + AF = (6 + x) cm
Now, s = `("a" + "b" + "c")/2`
= `(14 + (8 + "x") + (6 + "x"))/2`
= `(28 + 2"x")/2`
= (14 + x) cm
∴ Area of ΔABC = `sqrt("s"("s" - "a")("s" - "b")("s" - "c"))`
84 = `sqrt((14 + "x")(14 + "x" - 14)(14 + "x" - 8 - "x")(14 + "x" - 6 - "x"))`
84 = `sqrt("x"(14 + "x")(6)(8)`
84 = `sqrt(48"x"("x" + 14)) "cm"^2` ...(i)
`sqrt(48"x"("x" + 14))` = 84
On squaring both sides, we get
48x(x + 14) = 84 × 84
⇒ 4x(x + 14) = 84 × 7
⇒ x2 + 14x – 147 = 0
⇒ x2 + 21x – 7x – 147 = 0
x(x + 21) – 7(x + 21) = 0
(x + 21)(x – 7) = 0
So, x = 7, or x = –21 (rejected as - ve)
Hence, x = 7
Therefore, AB = c = 6 + x = 6 + 7 = 13 cm
AC = b = 8 + x = 8 + 7 = 15 cm
APPEARS IN
संबंधित प्रश्न
If from an external point P of a circle with centre O, two tangents PQ and PR are drawn such that ∠QPR = 120°, prove that 2PQ = PO.
Prove that the tangents drawn at the ends of a diameter of a circle are parallel.
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is ______.
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
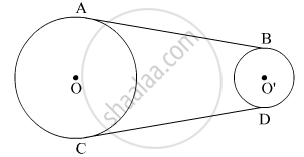
In the figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB = CD.
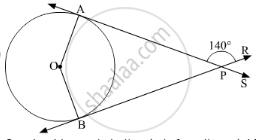
Find the angle between two radii at the centre of the circle as shown in the figure. Lines PA and PB are tangents to the circle at other ends of the radii and ∠APR = 140°.
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.

Find: ∠AOB
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
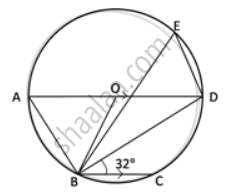
Find: ∠BED
The length of tangent from an external point P on a circle with centre O is always less than OP.
In the given figure, BC is tangent to the circle at point B of circle centred at O. BD is a chord of the circle so that ∠BAD = 55°. Find m∠DBC.

