Advertisements
Advertisements
प्रश्न
Prove that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
उत्तर

Given: Two tangents PQ and PR are drawn from an external points P to a circle with centre O.
To prove: Centre of a circle touching two intersecting lines lies on the angle bisector of angle formed by tangents.
Construction: Join OR and OQ.
In ∆POR and ∆POQ,
∠PRO = ∠PQO = 90° ...[Tangent at any point of a circle is perpendicular to the radius through the point of contact]
OR = OQ ...[Radii of same circle]
Since, OP is common
∴ ∆PRO ≅ ∆PQO ...[RHS]
Hence, ∠RPO = ∠QPO ...[By CPCT]
Thus, O lies on angle bisector of PR and PQ.
Hence proved.
संबंधित प्रश्न
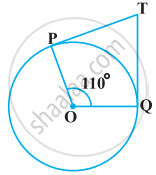
In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to ______.
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
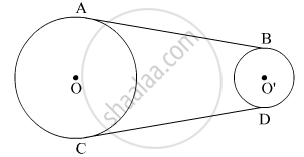
In the figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB = CD.
M and N are the midpoints of chords AB and CD . The line MN passes through the centre O . Prove that AB || CD.
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.

Find: ∠AOB
In Fig. 4, a circle is inscribed in a ΔABC having sides BC = 8 cm, AB = 10 cm and AC = 12 cm. Find the lengths BL, CM and AN.

The length of tangents drawn from an external point to the circle ______
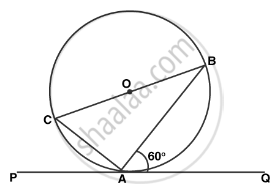
In the given figure, PAQ is the tangent. BC is the diameter of the circle. ∠BAQ = 60°, find ∠ABC.
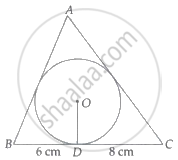
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.
From an external point, two tangents are drawn to a circle. Prove that the line joining the external point to the centre of the circle bisects the angle between the two tangents.
