Advertisements
Advertisements
प्रश्न
In the given figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
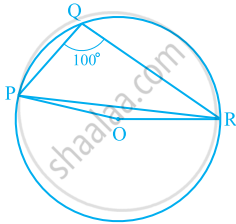
उत्तर

Consider PR as a chord of the circle.
Take any point S on the major arc of the circle.
PQRS is a cyclic quadrilateral.
∠PQR + ∠PSR = 180° ...(Opposite angles of a cyclic quadrilateral)
⇒ ∠PSR = 180° − 100° = 80°
We know that the angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.
∴ ∠POR = 2∠PSR = 2(80°) = 160°
In ΔPOR,
OP = OR ...(Radii of the same circle)
∴ ∠OPR = ∠ORP ...(Angles opposite to equal sides of a triangle)
∠OPR + ∠ORP + ∠POR = 180° ...(Angle sum property of a triangle)
2∠OPR + 160° = 180°
2∠OPR = 180° − 160° = 20°
∠OPR = 10°
APPEARS IN
संबंधित प्रश्न
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
Prove that the line of centres of two intersecting circles subtends equal angles at the two points of intersection.
Two congruent circles intersect each other at points A and B. Through A any line segment PAQ is drawn so that P, Q lie on the two circles. Prove that BP = BQ.
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
ABCD is a cyclic quadrilateral such that ∠ADB = 30° and ∠DCA = 80°, then ∠DAB =
If non-parallel sides of a trapezium are equal, prove that it is cyclic.
If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
The three angles of a quadrilateral are 100°, 60°, 70°. Find the fourth angle.
