Advertisements
Advertisements
प्रश्न
In the given triangle PQR, LM is parallel to QR and PM : MR = 3 : 4.
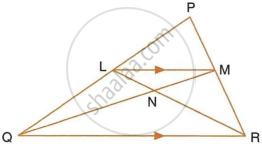
Calculate the value of ratio:
- `(PL)/(PQ)` and then `(LM)/(QR)`
- `"Area of ΔLMN"/"Area of ΔMNR"`
- `"Area of ΔLQM"/"Area of ΔLQN"`
उत्तर
i. In ∆PLM and ∆PQR,
As LM || QR, Corresponding angles are equal
∠PLM = ∠PQR
∠PML = ∠PRQ
∆PLM ~ ∆PQR
`=> 3/7 = (LM)/(QR)` ...`(∵ (PM)/(MR) = 3/4 => (PM)/(PR) = 3/7)`
Also, by using basic proportionality theorem, we have:
`(PL)/(LQ) = (PM)/(MR) = 3/4`
`=> (LQ)/(PL) = 4/3`
`=> 1 + (LQ)/(PL) = 1 + 4/3`
`=> (PL + LQ)/(PL) = (3 + 4)/3`
`=> (PQ)/(PL) = 7/3`
`=> (PL)/(PQ) = 3/7`
ii. Since ∆LMN and ∆MNR have common vertex at M and their bases LN and NR are along the same straight line
∴ `"Area of ΔLMN"/"Area of ΔMNR" = (LN)/(NR)`
Now, in ∆LNM and ∆RNQ
∠NLM = ∠NRQ ...(Alternate angles)
∠LMN = ∠NQR ...(Alternate angles)
∆LMN ~ ∆RNQ ...(AA Similarity)
∴ `(MN)/(QN) = (LN)/(NR) = (LM)/(QR) = 3/7`
∴ `"Area of ΔLMN"/"Area of ΔMNR" = (LN)/(NR) = 3/7`
iii. Since ΔLQM and ΔLQN have common vertex at L and their bases QM and QN are along the same straight line
`"Area of ΔLQM"/ "Area of ΔLQN" = (QM)/(QN) = 10/7`
`(∴ (NM)/(QN) = 3/7 => (QM)/(QN) = 10/7)`
APPEARS IN
संबंधित प्रश्न
In ∆ ABC, ∠B = 2 ∠C and the bisector of angle B meets CA at point D. Prove that:
(i) ∆ ABC and ∆ ABD are similar,
(ii) DC: AD = BC: AB
In ∆ABC, ∠B = 90° and BD ⊥ AC.
- If CD = 10 cm and BD = 8 cm; find AD.
- If AC = 18 cm and AD = 6 cm; find BD.
- If AC = 9 cm and AB = 7 cm; find AD.
Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting diagonal AC in L and AD produced in E. Prove that: EL = 2BL.
In the given figure, AX : XB = 3 : 5
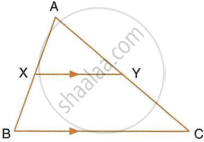
Find:
- the length of BC, if the length of XY is 18 cm.
- the ratio between the areas of trapezium XBCY and triangle ABC.
In the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1 : 2. DP produced meets AB produces at Q. Given the area of triangle CPQ = 20 cm2.

Calculate:
- area of triangle CDP,
- area of parallelogram ABCD.
The ratio between the altitudes of two similar triangles is 3 : 5; write the ratio between their :
- corresponding medians.
- perimeters.
- areas.
The ratio between the areas of two similar triangles is 16 : 25. State the ratio between their :
- perimeters.
- corresponding altitudes.
- corresponding medians.
In the following figure, AB, CD and EF are parallel lines. AB = 6cm, CD = y cm, EF = 10 cm, AC = 4 cm and CF = x cm. Calculate x and y 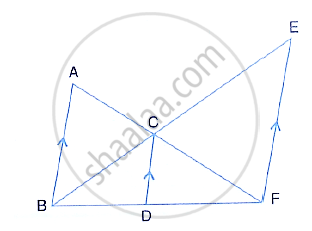
In ΔABC, ∠ACB = 90° and CD ⊥ AB.
Prove that : `(BC^2)/(AC^2)=(BD)/(AD)`
The following figure shows a triangle ABC in which AD and BE are perpendiculars to BC and AC respectively.
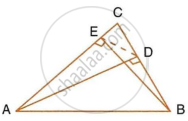
Show that:
- ΔADC ∼ ΔBEC
- CA × CE = CB × CD
- ΔABC ~ ΔDEC
- CD × AB = CA × DE
