Advertisements
Advertisements
प्रश्न
Let \[f\left( x \right) = x^2 and g\left( x \right) = 2^x\] Then, the solution set of the equation
पर्याय
R
{0}
{0, 2}
none of these
उत्तर
\[\text{Since}\left( \text{fog} \right)\left( x \right) = \left( \text{gof} \right)\left( x \right), \]
\[ f\left( g\left( x \right) \right) = g\left( f\left( x \right) \right)\]
\[ \Rightarrow f\left( 2^x \right) = g\left( x^2 \right)\]
\[ \Rightarrow \left( 2^x \right)^2 = 2^{x^2} \]
\[ \Rightarrow 2^{2x} = 2^{x^2} \]
\[ \Rightarrow x^2 = 2x\]
\[ \Rightarrow x^2 - 2x = 0\]
\[ \Rightarrow x\left( x - 2 \right) = 0\]
\[ \Rightarrow x = 0, 2\]
\[ \Rightarrow x \in \left\{ 0, 2 \right\}\]
So, the answer is (c) .
APPEARS IN
संबंधित प्रश्न
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x3
Show that the modulus function f: R → R given by f(x) = |x| is neither one-one nor onto, where |x| is x, if x is positive or 0 and |x| is − x if x is negative.
Show that the Signum Function f: R → R, given by `f(x) = {(1, if x > 0), (0, if x = 0), (-1, if x < 0):}` is neither one-one nor onto
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f: R → R defined by f(x) = 1 + x2
Let A and B be sets. Show that f: A × B → B × A such that (a, b) = (b, a) is bijective function.
Let A = {−1, 0, 1, 2}, B = {−4, −2, 0, 2} and f, g: A → B be functions defined by f(x) = x2 − x, x ∈ A and g(x) = `2|x - 1/2|- 1, x in A`. Are f and g equal?
Justify your answer. (Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) ∀ a ∈ A are called equal functions).
Show that the function f: ℝ → ℝ defined by f(x) = `x/(x^2 + 1), ∀x in R`is neither one-one nor onto. Also, if g: ℝ → ℝ is defined as g(x) = 2x - 1. Find fog(x)
Which of the following functions from A to B are one-one and onto?
f1 = {(1, 3), (2, 5), (3, 7)} ; A = {1, 2, 3}, B = {3, 5, 7}
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 5x3 + 4
Let A = [-1, 1]. Then, discuss whether the following function from A to itself is one-one, onto or bijective : `f (x) = x/2`
Let A = {a, b, c}, B = {u v, w} and let f and g be two functions from A to B and from B to A, respectively, defined as :
f = {(a, v), (b, u), (c, w)}, g = {(u, b), (v, a), (w, c)}.
Show that f and g both are bijections and find fog and gof.
Find fog (2) and gof (1) when : f : R → R ; f(x) = x2 + 8 and g : R → R; g(x) = 3x3 + 1.
Give examples of two functions f : N → N and g : N → N, such that gof is onto but f is not onto.
Give examples of two functions f : N → Z and g : Z → Z, such that gof is injective but gis not injective.
If f(x) = sin x and g(x) = 2x be two real functions, then describe gof and fog. Are these equal functions?
Let f : [−1, ∞) → [−1, ∞) be given by f(x) = (x + 1)2 − 1, x ≥ −1. Show that f is invertible. Also, find the set S = {x : f(x) = f−1 (x)}.
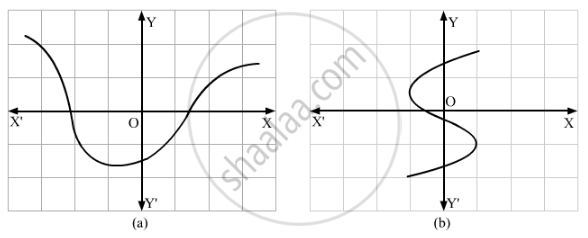
Which one of the following graphs represents a function?
If A = {a, b, c} and B = {−2, −1, 0, 1, 2}, write the total number of one-one functions from A to B.
If f(x) = x + 7 and g(x) = x − 7, x ∈ R, write fog (7).
Let f : R → R be the function defined by f(x) = 4x − 3 for all x ∈ R Then write f . [NCERT EXEMPLAR]
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
Let
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = B\] Then, the mapping\[f : A \to \text{B given by} f\left( x \right) = x\left| x \right|\] is
Let
\[f : R - \left\{ n \right\} \to R\]
If \[f : R \to R is given by f\left( x \right) = 3x - 5, then f^{- 1} \left( x \right)\]
Show that the function f: R → R defined by f(x) = `x/(x^2 + 1)`, ∀ ∈ + R , is neither one-one nor onto
Let D be the domain of the real valued function f defined by f(x) = `sqrt(25 - x^2)`. Then, write D
Let f: R → R be the function defined by f(x) = 2x – 3 ∀ x ∈ R. write f–1
Let C be the set of complex numbers. Prove that the mapping f: C → R given by f(z) = |z|, ∀ z ∈ C, is neither one-one nor onto.
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
f = {(1, 4), (1, 5), (2, 4), (3, 5)}
Let A = {1, 2, 3, ...n} and B = {a, b}. Then the number of surjections from A into B is ______.
Let f: R – `{3/5}` → R be defined by f(x) = `(3x + 2)/(5x - 3)`. Then ______.
The function f : A → B defined by f(x) = 4x + 7, x ∈ R is ____________.
Let g(x) = x2 – 4x – 5, then ____________.
Let R be a relation on the set L of lines defined by l1 R l2 if l1 is perpendicular to l2, then relation R is ____________.
Let f: R → R defined by f(x) = x4. Choose the correct answer
Function f: R → R, defined by f(x) = `x/(x^2 + 1)` ∀ x ∈ R is not
Let f(n) = `[1/3 + (3n)/100]n`, where [n] denotes the greatest integer less than or equal to n. Then `sum_(n = 1)^56f(n)` is equal to ______.
If f : R `rightarrow` R is defined by `f(x) = (2x - 7)/4`, show that f(x) is one-one and onto.
