Advertisements
Advertisements
प्रश्न
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
उत्तर
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Injectivity:
f2 (2) = a
f2 (3) = b
f2 (4) = c
⇒ Every element of A has different images in B.
So, f2 is one-one.
Surjectivity:
Co-domain of f2 = {a, b, c}
Range of f2 = set of images = {a, b, c}
⇒ Co-domain = range
So, f2 is onto.
APPEARS IN
संबंधित प्रश्न
Show that the function f in `A=R-{2/3} ` defined as `f(x)=(4x+3)/(6x-4)` is one-one and onto hence find f-1
Show that the Signum Function f: R → R, given by `f(x) = {(1, if x > 0), (0, if x = 0), (-1, if x < 0):}` is neither one-one nor onto
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f: R → R defined by f(x) = 1 + x2
Find the number of all onto functions from the set {1, 2, 3, …, n} to itself.
Let A = {−1, 0, 1, 2}, B = {−4, −2, 0, 2} and f, g: A → B be functions defined by f(x) = x2 − x, x ∈ A and g(x) = `2|x - 1/2|- 1, x in A`. Are f and g equal?
Justify your answer. (Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) ∀ a ∈ A are called equal functions).
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Classify the following function as injection, surjection or bijection :
f : Q − {3} → Q, defined by `f (x) = (2x +3)/(x-3)`
Classify the following function as injection, surjection or bijection :
f : Q → Q, defined by f(x) = x3 + 1
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 3 − 4x
Let A = [-1, 1]. Then, discuss whether the following functions from A to itself is one-one, onto or bijective : h(x) = x2
If A = {1, 2, 3}, show that a onto function f : A → A must be one-one.
Suppose f1 and f2 are non-zero one-one functions from R to R. Is `f_1 / f^2` necessarily one - one? Justify your answer. Here,`f_1/f_2 : R → R is given by (f_1/f_2) (x) = (f_1(x))/(f_2 (x)) for all x in R .`
Let f : R → R and g : R → R be defined by f(x) = x2 and g(x) = x + 1. Show that fog ≠ gof.
Find fog and gof if : f (x) = x2 g(x) = cos x .
Find fog and gof if : f(x) = `x^2` + 2 , g (x) = 1 − `1/ (1-x)`.
Find f −1 if it exists : f : A → B, where A = {0, −1, −3, 2}; B = {−9, −3, 0, 6} and f(x) = 3 x.
Let f : R `{- 4/3} `- 43 →">→ R be a function defined as f(x) = `(4x)/(3x +4)` . Show that f : R - `{-4/3}`→ Rang (f) is one-one and onto. Hence, find f -1.
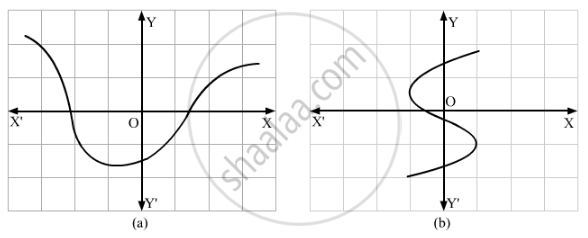
Which one of the following graphs represents a function?
If f : C → C is defined by f(x) = x2, write f−1 (−4). Here, C denotes the set of all complex numbers.
If f : R → R is defined by f(x) = x2, find f−1 (−25).
Let \[f : \left( - \frac{\pi}{2}, \frac{\pi}{2} \right) \to R\] be a function defined by f(x) = cos [x]. Write range (f).
Let f : R → R, g : R → R be two functions defined by f(x) = x2 + x + 1 and g(x) = 1 − x2. Write fog (−2).
Let A = {a, b, c, d} and f : A → A be given by f = {( a,b ),( b , d ),( c , a ) , ( d , c )} write `f^-1`. [NCERT EXEMPLAR]
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
Which of the following functions form Z to itself are bijections?
\[f : Z \to Z\] be given by
` f (x) = {(x/2, ", if x is even" ) ,(0 , ", if x is odd "):}`
Then, f is
If \[g\left( x \right) = x^2 + x - 2\text{ and} \frac{1}{2} gof\left( x \right) = 2 x^2 - 5x + 2\] is equal to
Mark the correct alternative in the following question:
Let A = {1, 2, ... , n} and B = {a, b}. Then the number of subjections from A into B is
Let f, g: R → R be two functions defined as f(x) = |x| + x and g(x) = x – x ∀ x ∈ R. Then, find f o g and g o f
Given a function If as f(x) = 5x + 4, x ∈ R. If g : R → R is inverse of function ‘f then
A general election of Lok Sabha is a gigantic exercise. About 911 million people were eligible to vote and voter turnout was about 67%, the highest ever

Let I be the set of all citizens of India who were eligible to exercise their voting right in the general election held in 2019. A relation ‘R’ is defined on I as follows:
R = {(V1, V2) ∶ V1, V2 ∈ I and both use their voting right in the general election - 2019}
- Mr. ’X’ and his wife ‘W’ both exercised their voting right in the general election-2019, Which of the following is true?
A function f: x → y is/are called onto (or surjective) if x under f.
Consider a function f: `[0, pi/2] ->` R, given by f(x) = sinx and `g[0, pi/2] ->` R given by g(x) = cosx then f and g are
The solution set of the inequation log1/3(x2 + x + 1) + 1 > 0 is ______.
Difference between the greatest and least value of f(x) = `(1 + (cos^-1x)/π)^2 - (1 + (sin^-1x)/π)^2` is ______.
Let S = {1, 2, 3, 4, 5, 6, 7}. Then the number of possible functions f: S `rightarrow` S such that f(m.n) = f(m).f(n) for every m, n ∈ S and m.n ∈ S is equal to ______.
Let A = {1, 2, 3, ..., 10} and f : A `rightarrow` A be defined as
f(k) = `{{:(k + 1, if k "is odd"),( k, if k "is even"):}`.
Then the number of possible functions g : A `rightarrow` A such that gof = f is ______.
