Advertisements
Advertisements
Question
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Solution
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Injectivity:
f2 (2) = a
f2 (3) = b
f2 (4) = c
⇒ Every element of A has different images in B.
So, f2 is one-one.
Surjectivity:
Co-domain of f2 = {a, b, c}
Range of f2 = set of images = {a, b, c}
⇒ Co-domain = range
So, f2 is onto.
APPEARS IN
RELATED QUESTIONS
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f : R → R defined by f(x) = 3 − 4x
Let f: R → R be defined as f(x) = 3x. Choose the correct answer.
Classify the following function as injection, surjection or bijection : f : N → N given by f(x) = x3
Let A = [-1, 1]. Then, discuss whether the following function from A to itself is one-one, onto or bijective : g(x) = |x|
Find gof and fog when f : R → R and g : R → R is defined by f(x) = x and g(x) = |x| .
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 8x3 and g(x) = x1/3.
Let f = {(3, 1), (9, 3), (12, 4)} and g = {(1, 3), (3, 3) (4, 9) (5, 9)}. Show that gof and fog are both defined. Also, find fog and gof.
Consider f : N → N, g : N → N and h : N → R defined as f(x) = 2x, g(y) = 3y + 4 and h(z) = sin z for all x, y, z ∈ N. Show that ho (gof) = (hog) of.
Find fog and gof if : f (x) = x2 g(x) = cos x .
Let f be a function from R to R, such that f(x) = cos (x + 2). Is f invertible? Justify your answer.
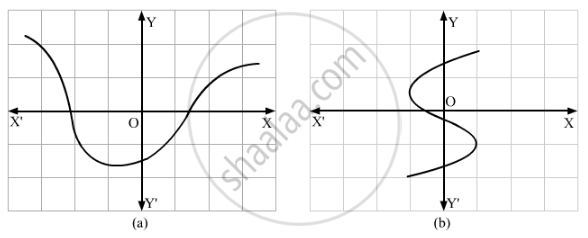
Which one of the following graphs represents a function?
If f : R → R is defined by f(x) = x2, write f−1 (25)
Let C denote the set of all complex numbers. A function f : C → C is defined by f(x) = x3. Write f−1(1).
Let \[f : \left( - \frac{\pi}{2}, \frac{\pi}{2} \right) \to R\] be a function defined by f(x) = cos [x]. Write range (f).
Let f : R → R, g : R → R be two functions defined by f(x) = x2 + x + 1 and g(x) = 1 − x2. Write fog (−2).
Let f be an invertible real function. Write ( f-1 of ) (1) + ( f-1 of ) (2) +..... +( f-1 of ) (100 )
Write the domain of the real function
`f (x) = sqrtx - [x] .`
Let the function
\[f : R - \left\{ - b \right\} \to R - \left\{ 1 \right\}\]
\[f\left( x \right) = \frac{x + a}{x + b}, a \neq b .\text{Then},\]
Let \[f\left( x \right) = \frac{1}{1 - x} . \text{Then}, \left\{ f o \left( fof \right) \right\} \left( x \right)\]
The distinct linear functions that map [−1, 1] onto [0, 2] are
If \[f\left( x \right) = \sin^2 x\] and the composite function \[g\left( f\left( x \right) \right) = \left| \sin x \right|\] then g(x) is equal to
Mark the correct alternative in the following question:
If the set A contains 7 elements and the set B contains 10 elements, then the number one-one functions from A to B is
Let A = R − (2) and B = R − (1). If f: A ⟶ B is a function defined by`"f(x)"=("x"-1)/("x"-2),` how that f is one-one and onto. Hence, find f−1.
Write about strlen() function.
Let A be a finite set. Then, each injective function from A into itself is not surjective.
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(x, y): x is a person, y is the mother of x}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
f = {(1, 4), (1, 5), (2, 4), (3, 5)}
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
g(x) = |x|
Let f: R → R be the functions defined by f(x) = x3 + 5. Then f–1(x) is ______.
The smallest integer function f(x) = [x] is ____________.
Let f : R → R be a function defined by f(x) `= ("e"^abs"x" - "e"^-"x")/("e"^"x" + "e"^-"x")` then f(x) is
Let f : [0, ∞) → [0, 2] be defined by `"f" ("x") = (2"x")/(1 + "x"),` then f is ____________.
If `f : R -> R^+ U {0}` be defined by `f(x) = x^2, x ∈ R`. The mapping is
If log102 = 0.3010.log103 = 0.4771 then the number of ciphers after decimal before a significant figure comes in `(5/3)^-100` is ______.
The function f(x) = [x], where [x] denotes the greatest integer less than or equal to x; is continuous at ______.
Which one of the following graphs is a function of x?
 |
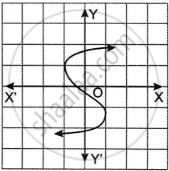 |
| Graph A | Graph B |
